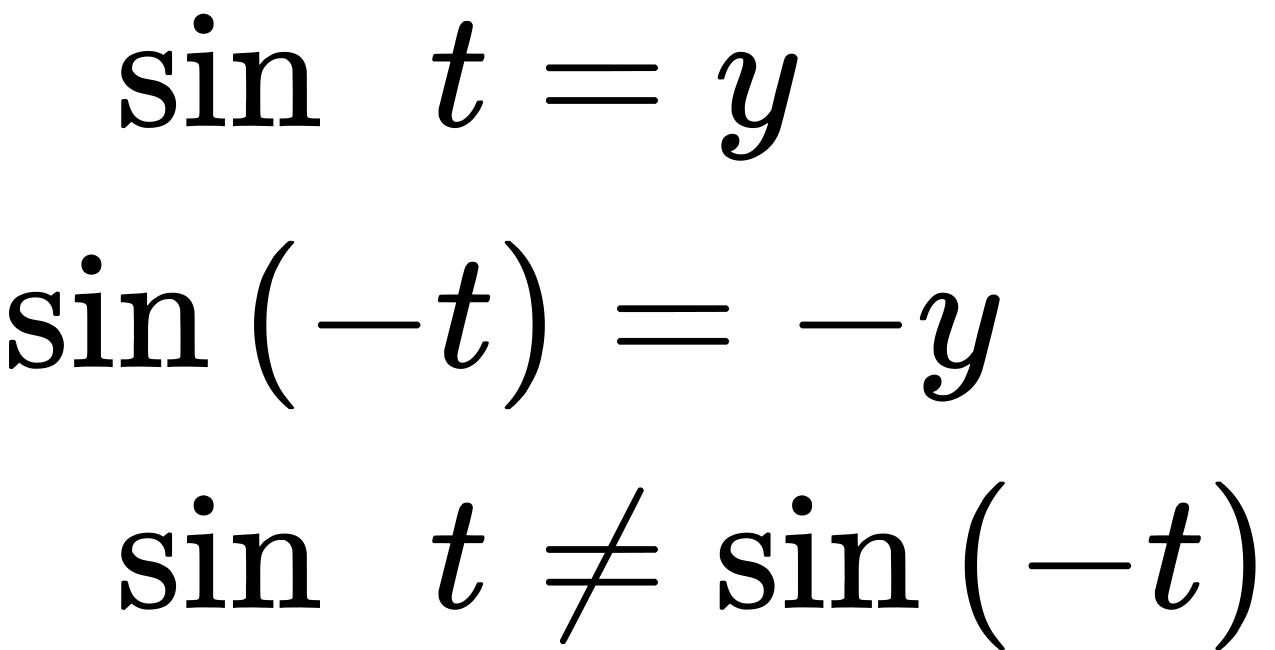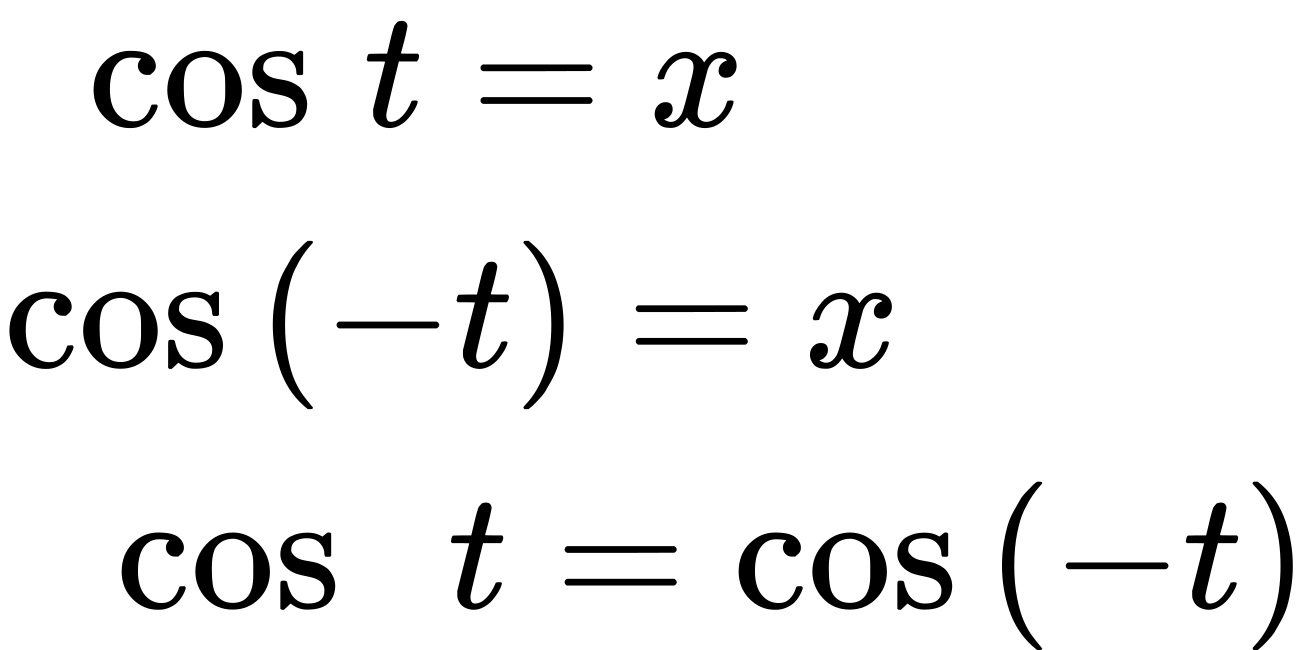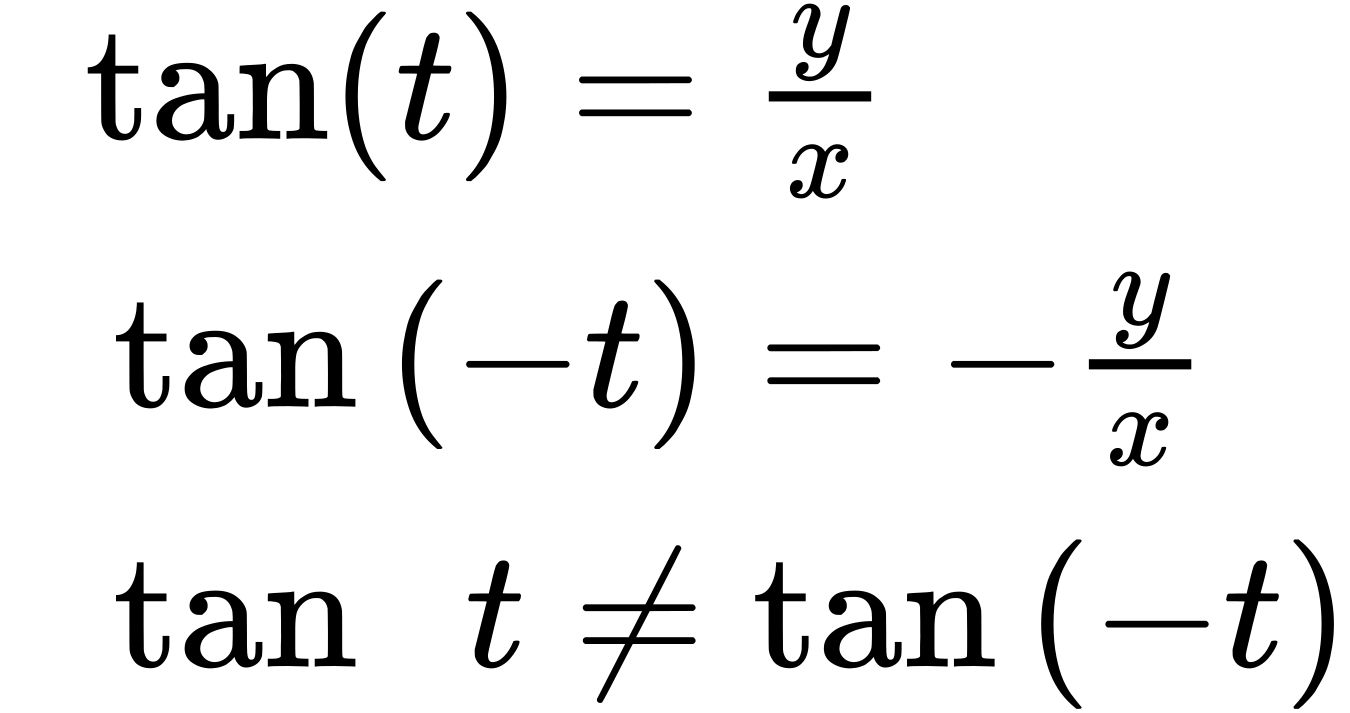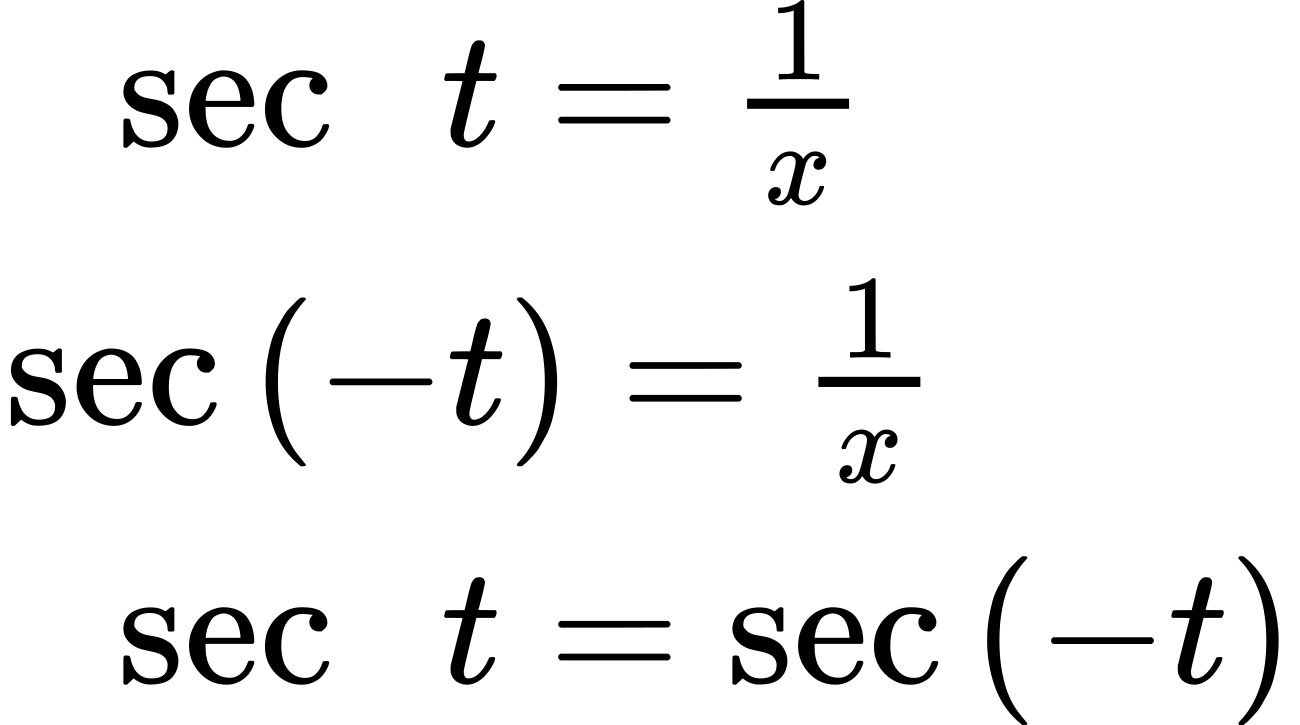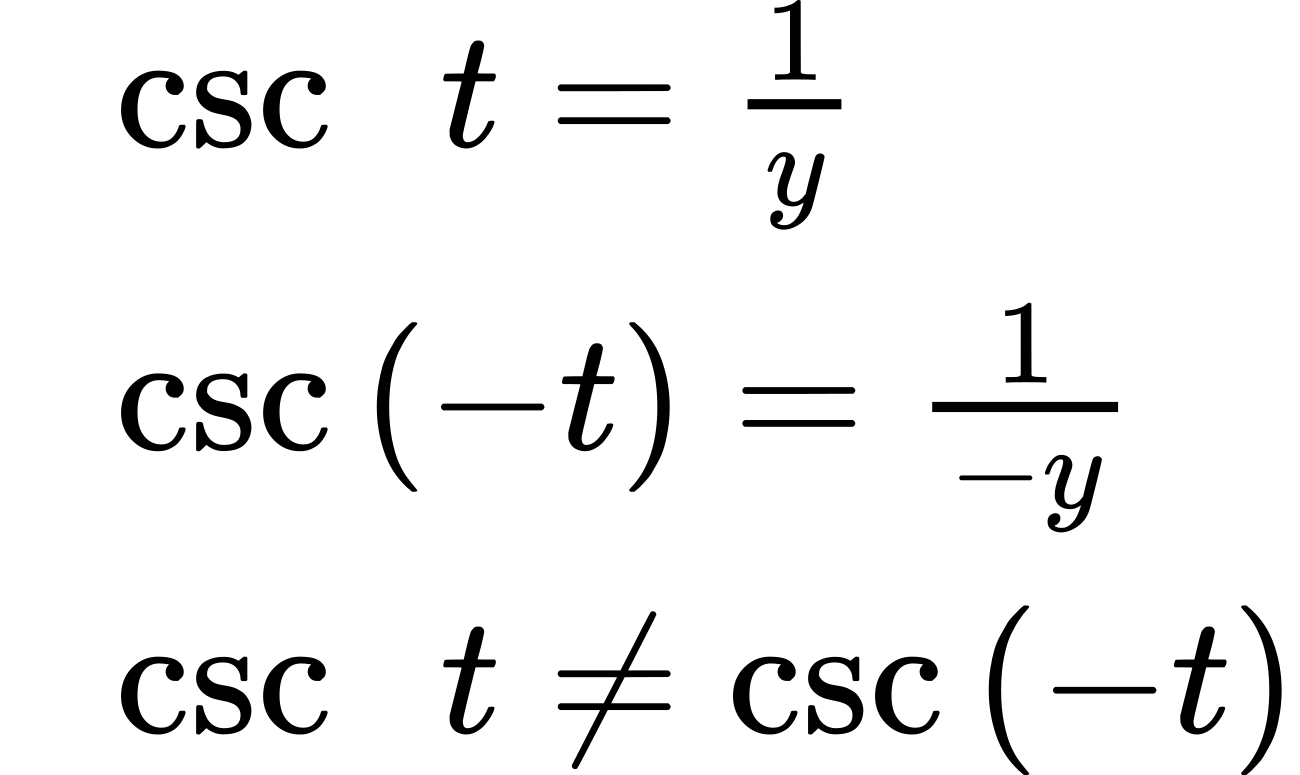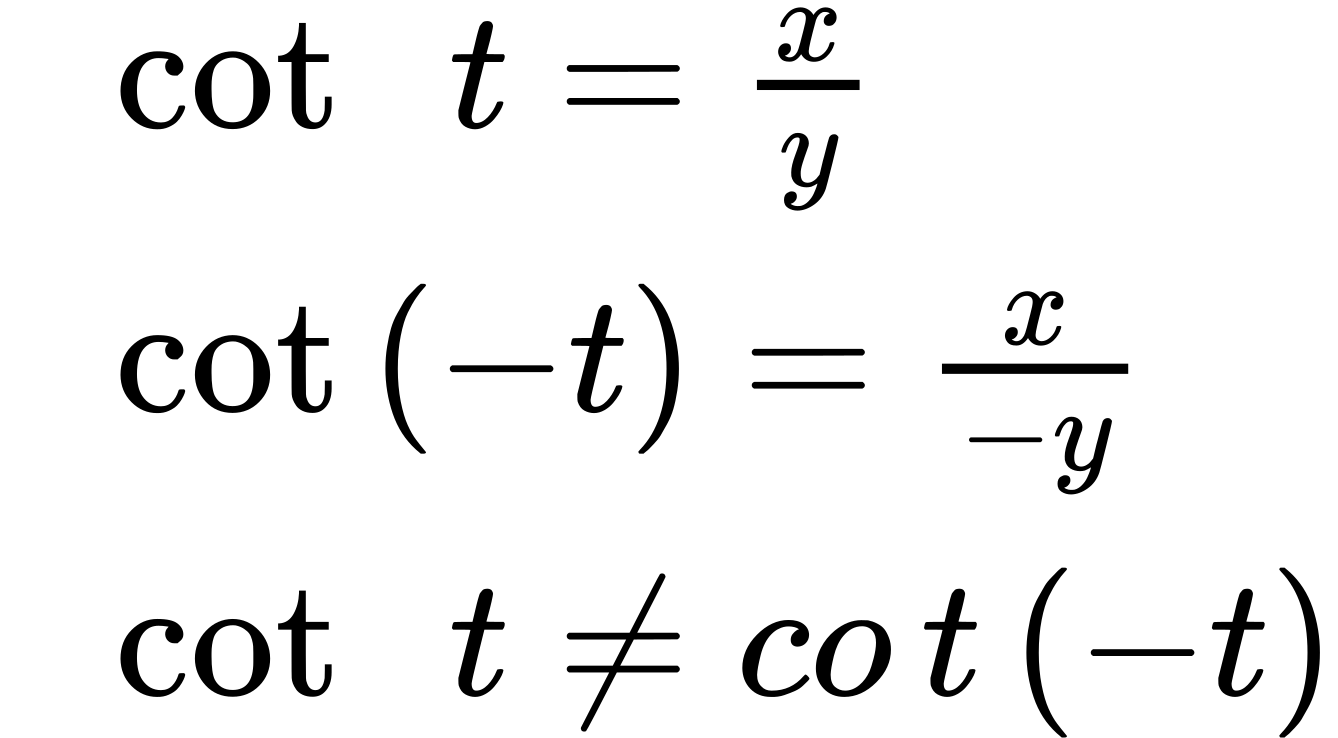Section 2.3 – The Other Trigonometric Functions
Learning Objectives
Welcome to Section 2.3! In this section, you will…
- Find exact values of the trigonometric functions secant, cosecant, tangent, and cotangent of [latex]\frac{π}{3}[/latex], [latex]\frac{π}{4}[/latex], and [latex]\frac{π}{6}[/latex]
- Use reference angles to evaluate the trigonometric functions secant, cosecant, tangent, and cotangent.
- Use properties of even and odd trigonometric functions.
- Recognize and use fundamental identities.
- Evaluate trigonometric functions with a calculator.
A wheelchair ramp that meets the standards of the Americans with Disabilities Act must make an angle with the ground whose tangent is [latex]\frac{1}{12}[/latex] or less, regardless of its length. A tangent represents a ratio, so this means that for every 1 inch of rise, the ramp must have 12 inches of run. Trigonometric functions allow us to specify the shapes and proportions of objects independent of exact dimensions. We have already defined the sine and cosine functions of an angle. Though sine and cosine are the trigonometric functions most often used, there are four others. Together they make up the set of six trigonometric functions. In this section, we will investigate the remaining functions.
Finding Exact Values of the Trigonometric Functions Secant, Cosecant, Tangent, and Cotangent
To define the remaining functions, we will once again draw a unit circle with a point [latex](x,y)[/latex] corresponding to an angle of [latex]t[/latex], as shown in Figure 1. As with the sine and cosine, we can use the [latex](x,y)[/latex] coordinates to find the other functions.
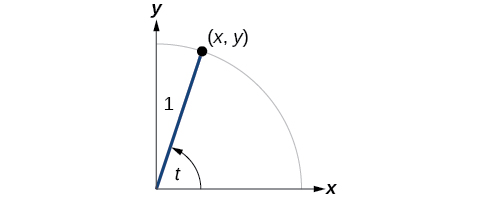
The first function we will define is the tangent. The tangent of an angle is the ratio of the y–value to the x–value of the corresponding point on the unit circle. In Figure 1, the tangent of angle [latex]t[/latex] is equal to [latex]\frac{y}{x}[/latex], [latex]x[/latex] ≠ 0. Because the y–value is equal to the sine of [latex]t[/latex], and the x–value is equal to the cosine of [latex]t[/latex], the tangent of angle [latex]t[/latex] can also be defined as [latex]\frac{sin t}{cos t}[/latex], [latex]cos t[/latex] ≠ 0. The tangent function is abbreviated as [latex]tan[/latex]. The remaining three functions can all be expressed as reciprocals of functions we have already defined.
- The secant function is the reciprocal of the cosine function. In Figure 1, the secant of angle [latex]t[/latex] is equal to [latex]\frac{1}{cos t}[/latex] = [latex]\frac{1}{x}[/latex], [latex]x[/latex] ≠ 0. The secant function is abbreviated as [latex]sec[/latex].
- The cotangent function is the reciprocal of the tangent function. In Figure 1, the cotangent of angle [latex]t[/latex] is equal to [latex]\frac{cos t}{sin t}[/latex] = [latex]\frac{x}{y}[/latex], [latex]y[/latex] ≠ 0. The cotangent function is abbreviated as [latex]cot[/latex].
- The cosecant function is the reciprocal of the sine function. In Figure 1, the cosecant of angle [latex]t[/latex] is equal to [latex]\frac{1}{sin t}[/latex] = [latex]\frac{1}{y}[/latex], [latex]y[/latex] ≠ 0. The cosecant function is abbreviated as [latex]csc[/latex].
Tangent, Secant, Cosecant, and Cotangent Functions
If [latex]t[/latex] is a real number and [latex](x,y)[/latex] is a point where the terminal side of an angle of [latex]t[/latex] radians intercepts the unit circle, then

Example 1
Finding Trigonometric Functions from a Point on the Unit Circle
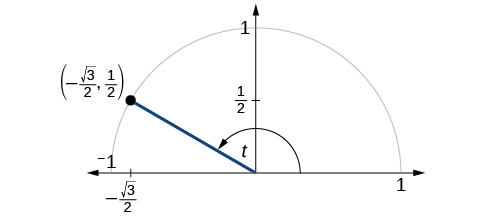
The point (-[latex]\frac{\sqrt{3}}{2}[/latex], [latex]\frac{1}{2}[/latex]) is on the unit circle, as shown in Figure 2. Find [latex]sin t[/latex], [latex]cos t[/latex], [latex]tan t[/latex], [latex]sec t[/latex], [latex]csc t[/latex], and [latex]cot t[/latex].
Show/Hide Solution
Solution
Because we know the [latex](x,y)[/latex] coordinates of the point on the unit circle indicated by angle [latex]t[/latex], we can use those coordinates to find the six functions:
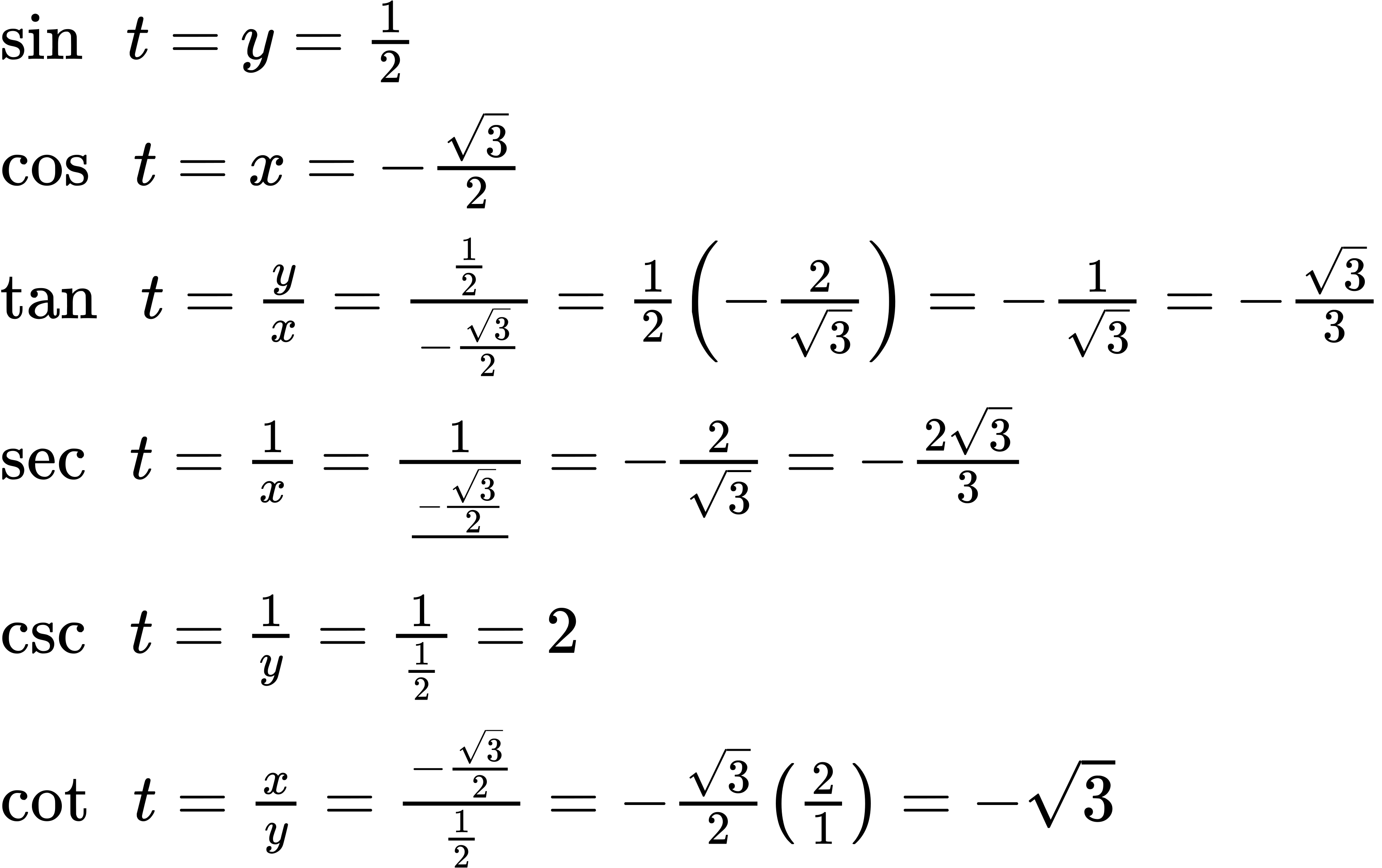
Try It #1
The point ([latex]\frac{\sqrt{2}}{2}[/latex], –[latex]\frac{\sqrt{2}}{2}[/latex]) is on the unit circle, as shown in Figure 3. Find [latex]sin t[/latex], [latex]cos t[/latex], [latex]tan t[/latex], [latex]sec t[/latex], [latex]csc t[/latex], and [latex]cot t[/latex].

Example 2
Finding the Trigonometric Functions of an Angle
Find [latex]sin t[/latex], [latex]cos t[/latex], [latex]tan t[/latex], [latex]sec t[/latex], [latex]csc t[/latex], and [latex]cot t[/latex] when [latex]t[/latex] = [latex]\frac{π}{6}[/latex].
Show/Hide Solution
Solution
We have previously used the properties of equilateral triangles to demonstrate that [latex]sin[/latex] [latex]\frac{π}{6}[/latex] = [latex]\frac{1}{2}[/latex] and [latex]cos[/latex] [latex]\frac{π}{6}[/latex] = [latex]\frac{\sqrt{3}}{2}[/latex]. We can use these values and the definitions of tangent, secant, cosecant, and cotangent as functions of sine and cosine to find the remaining function values.
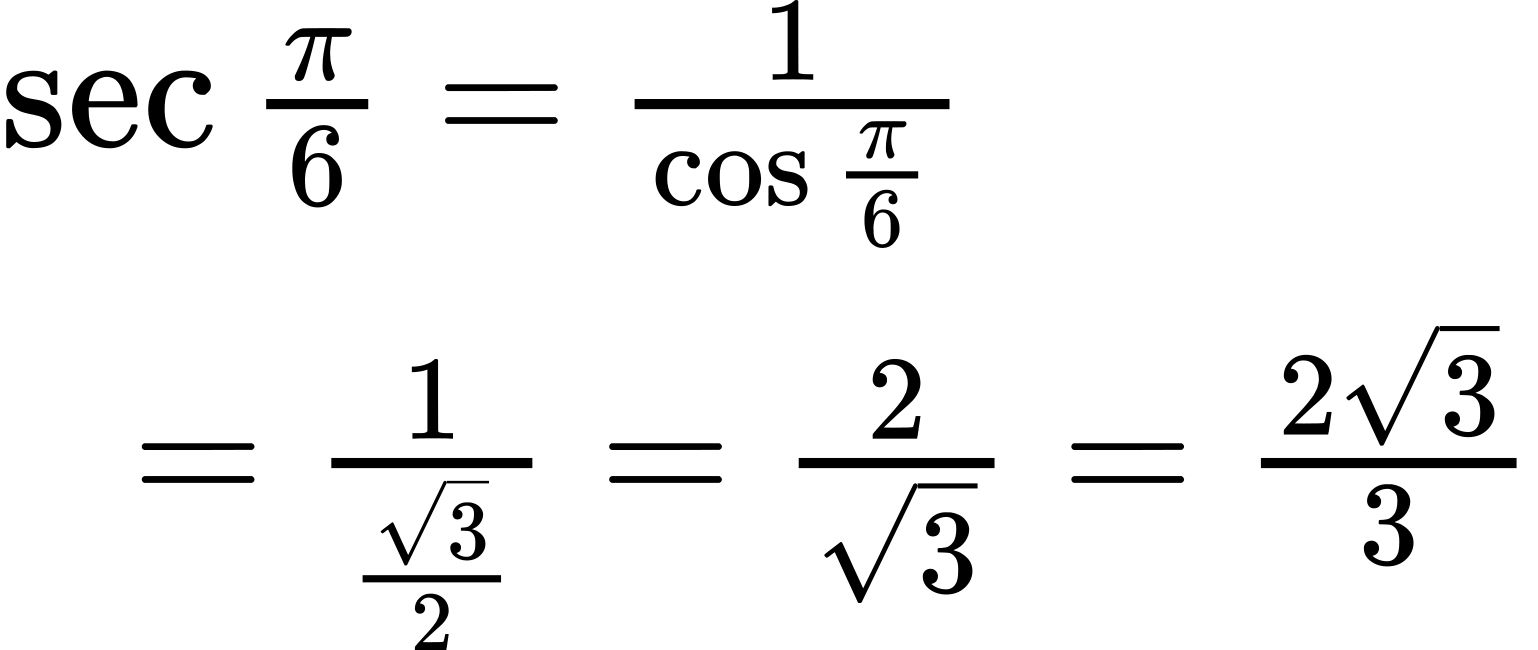
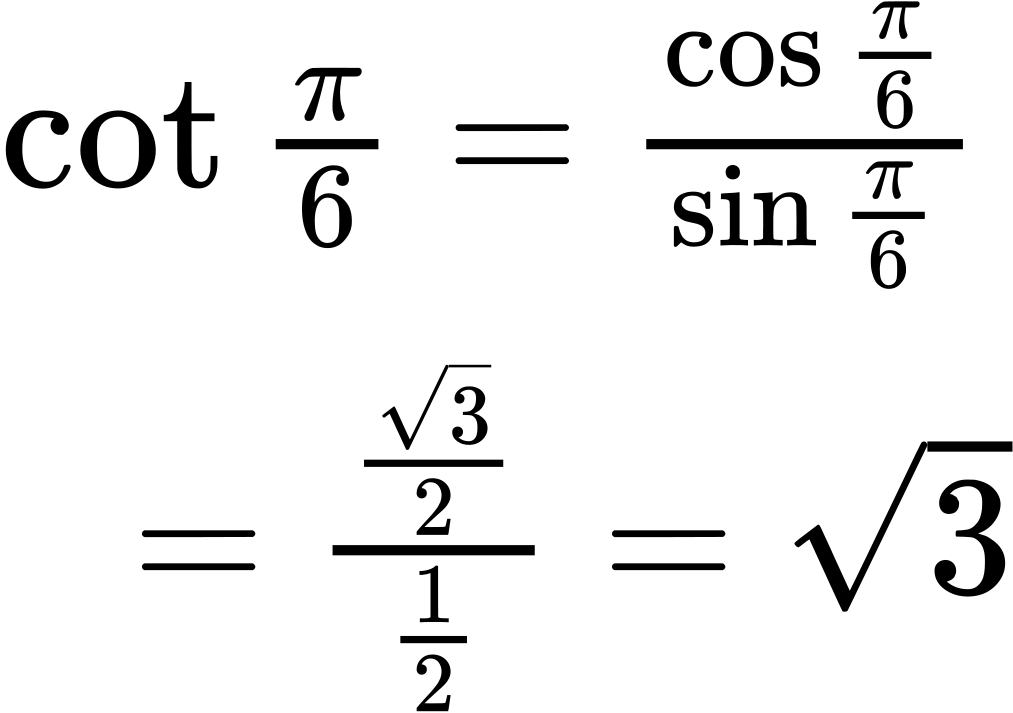
Try It #2
Find [latex]sin t[/latex], [latex]cos t[/latex], [latex]tan t[/latex], [latex]sec t[/latex], [latex]csc t[/latex], and [latex]cot t[/latex] when [latex]t[/latex] = [latex]\frac{π}{3}[/latex].
Because we know the sine and cosine values for the common first-quadrant angles, we can find the other function values for those angles as well by setting [latex]x[/latex] equal to the cosine and [latex]y[/latex] equal to the sine and then using the definitions of tangent, secant, cosecant, and cotangent. The results are shown in Table 1.
|
Angle |
0
|
[latex]\frac{π}{6}[/latex], or 30°
|
[latex]\frac{π}{4}[/latex], or 45°
|
[latex]\frac{π}{3}[/latex], or 60°
|
[latex]\frac{π}{2}[/latex], or 90°
|
|
Cosine |
1 |
[latex]\frac{\sqrt{3}}{2}[/latex] | [latex]\frac{\sqrt{2}}{2}[/latex] | [latex]\frac{1}{2}[/latex] |
0 |
|
Sine |
0 |
[latex]\frac{1}{2}[/latex] | [latex]\frac{\sqrt{2}}{2}[/latex] | [latex]\frac{\sqrt{3}}{2}[/latex] |
1 |
|
Tangent |
0 |
[latex]\frac{\sqrt{3}}{3}[/latex] |
1 |
[latex]\sqrt{3}[/latex] |
Undefined |
|
Secant |
1 |
[latex]\frac{2\sqrt{3}}{3}[/latex] | [latex]\sqrt{2}[/latex] |
2 |
Undefined |
|
Cosecant |
Undefined |
2 |
[latex]\sqrt{2}[/latex] | [latex]\frac{2\sqrt{3}}{3}[/latex] |
1 |
|
Cotangent |
Undefined |
[latex]\sqrt{3}[/latex] |
1 |
[latex]\frac{\sqrt{3}}{3}[/latex] |
0 |
Using Reference Angles to Evaluate Tangent, Secant, Cosecant, and Cotangent
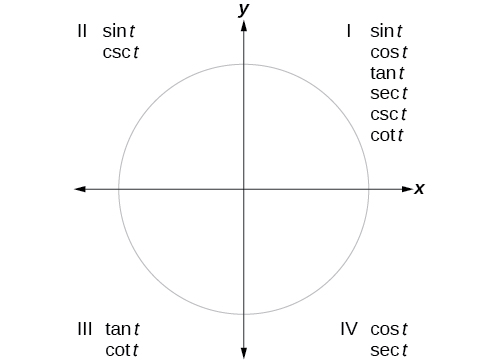
We can evaluate trigonometric functions of angles outside the first quadrant using reference angles as we have already done with the sine and cosine functions. The procedure is the same: Find the reference angle formed by the terminal side of the given angle with the horizontal axis. The trigonometric function values for the original angle will be the same as those for the reference angle, except for the positive or negative sign, which is determined by x– and y-values in the original quadrant. Figure 4 shows which functions are positive in which quadrant.
To help us remember which of the six trigonometric functions are positive in each quadrant, we can use the mnemonic phrase “A Smart Trig Class.” Each of the four words in the phrase corresponds to one of the four quadrants, starting with quadrant I and rotating counterclockwise. In quadrant I, which is “A,” all of the six trigonometric functions are positive. In quadrant II, “Smart,” only sine and its reciprocal function, cosecant, are positive. In quadrant III, “Trig,” only tangent and its reciprocal function, cotangent, are positive. Finally, in quadrant IV, “Class,” only cosine and its reciprocal function, secant, are positive.
How To
Given an angle not in the first quadrant, use reference angles to find all six trigonometric functions.
- Measure the angle formed by the terminal side of the given angle and the horizontal axis. This is the reference angle.
- Evaluate the function at the reference angle.
- Observe the quadrant where the terminal side of the original angle is located. Based on the quadrant, determine whether the output is positive or negative.
Example 3
Using Reference Angles to Find Trigonometric Functions
Use reference angles to find all six trigonometric functions of –[latex]\frac{5π}{6}[/latex].
Show/Hide Solution
Solution
The angle between this angle’s terminal side and the x–axis is [latex]\frac{π}{6}[/latex], so that is the reference angle. Since –[latex]\frac{5π}{6}[/latex] is in the third quadrant, where both [latex]x[/latex] and [latex]y[/latex] are negative, cosine, sine, secant, and cosecant will be negative, while tangent and cotangent will be positive.
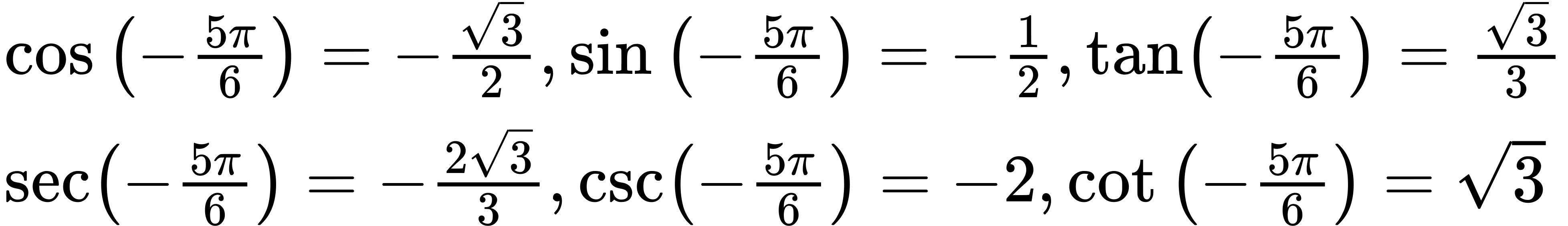
Try It #3
Use reference angles to find all six trigonometric functions of –[latex]\frac{7π}{4}[/latex].
Using Even and Odd Trigonometric Functions
To be able to use our six trigonometric functions freely with both positive and negative angle inputs, we should examine how each function treats a negative input. As it turns out, there is an important difference among the functions in this regard.
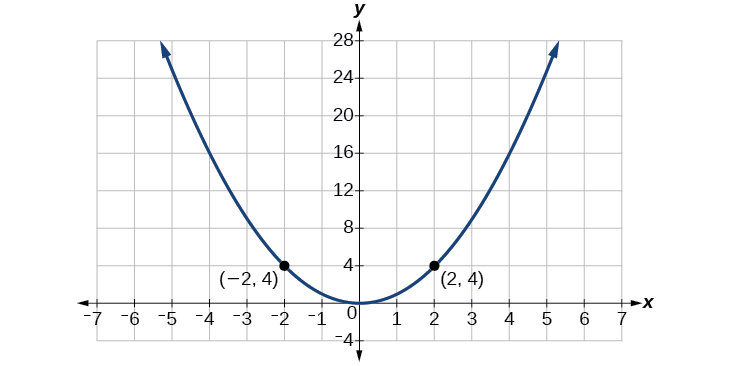
Consider the function [latex]f(x)[/latex] = [latex]x^2[/latex], shown in Figure 5. The graph of the function is symmetrical about the y–axis. All along the curve, any two points with opposite x–values have the same function value. This matches the result of calculation: [latex](4)^2[/latex] = [latex](−4)^2[/latex], [latex](−5)^2[/latex] = [latex](5)^2[/latex], and so on. So [latex]f(x)[/latex] = [latex]x^2[/latex] is an even function, a function such that two inputs that are opposites have the same output. That means [latex]f(−x)[/latex] = [latex]f(x)[/latex].
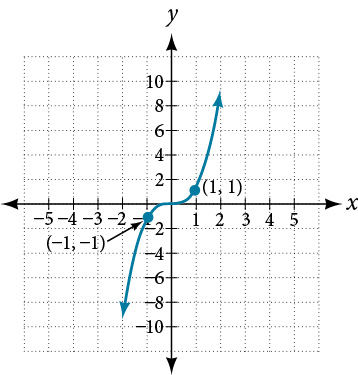
Now consider the function [latex]f(x)[/latex] = [latex]x^3[/latex], shown in Figure 6. The graph is not symmetrical about the y–axis. All along the graph, any two points with opposite x–values also have opposite y–values. So [latex]f(x)[/latex] = [latex]x^3[/latex] is an odd function, one such that two inputs that are opposites have outputs that are also opposites. That means [latex]f(−x)[/latex] = –[latex]f(x)[/latex].
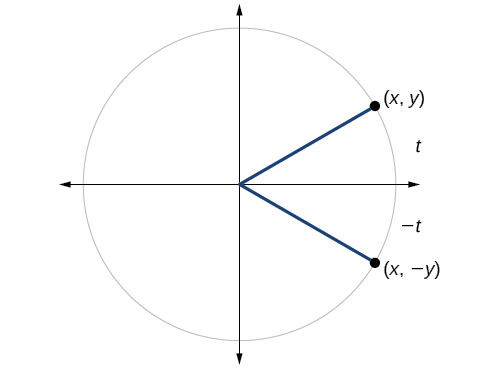
We can test whether a trigonometric function is even or odd by drawing a unit circle with a positive and a negative angle, as in Figure 7. The sine of the positive angle is [latex]y[/latex]. The sine of the negative angle is −[latex]y[/latex]. The sine function, then, is an odd function. We can test each of the six trigonometric functions in this fashion. The results are shown in Table 2.
|
|
|
|
|
|
|
|
Even and Odd Trigonometric Functions
An even function is one in which [latex]f(−x)[/latex] = [latex]f(x)[/latex].
An odd function is one in which [latex]f(−x)[/latex] = –[latex]f(x)[/latex].
Cosine and secant are even:

Sine, tangent, cosecant, and cotangent are odd:
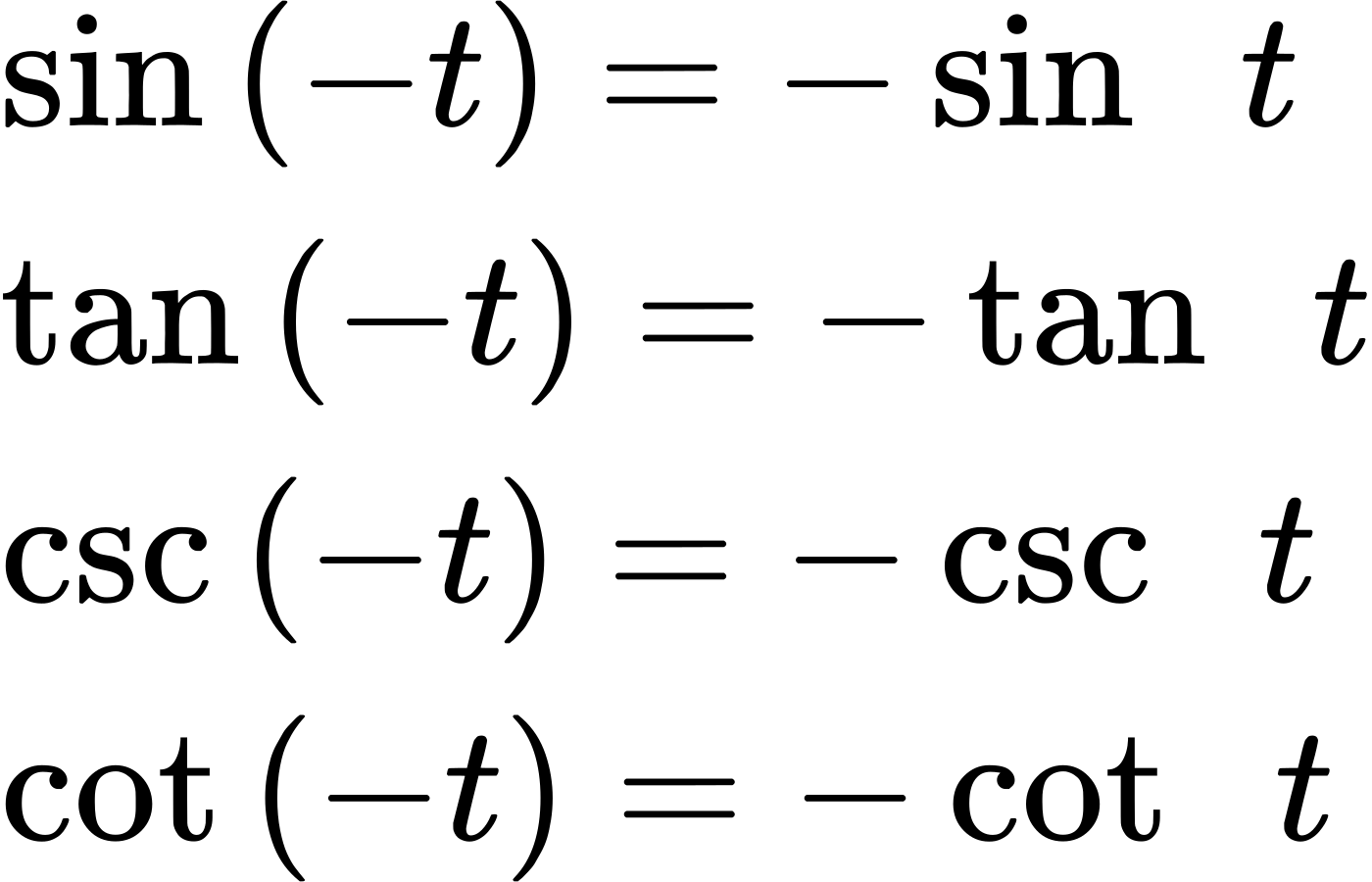
Example 4
Using Even and Odd Properties of Trigonometric Functions
If the secant of angle [latex]t[/latex] is 2, what is the secant of −[latex]t[/latex]?
Show/Hide Solution
Solution
Secant is an even function. The secant of an angle is the same as the secant of its opposite. So if the secant of angle [latex]t[/latex] is 2, the secant of −[latex]t[/latex] is also 2.
Try It #4
If the cotangent of angle [latex]t[/latex] is [latex]\sqrt{3}[/latex], what is the cotangent of −[latex]t[/latex]?
Recognizing and Using Fundamental Identities
We have explored a number of properties of trigonometric functions. Now, we can take the relationships a step further, and derive some fundamental identities. Identities are statements that are true for all values of the input on which they are defined. Usually, identities can be derived from definitions and relationships we already know. For example, the Pythagorean Identity we learned earlier was derived from the Pythagorean Theorem and the definitions of sine and cosine.
Fundamental Identities
We can derive some useful identities from the six trigonometric functions. The other four trigonometric functions can be related back to the sine and cosine functions using these basic relationships:
[latex]tan t[/latex] = [latex]\dfrac{sin t}{cos t}[/latex]
[latex]sec t[/latex] = [latex]\dfrac{1}{cos t}[/latex]
[latex]csc t[/latex] =[latex]\dfrac{1}{sin t}[/latex]
[latex]cot t[/latex] = [latex]\dfrac{1}{tan t}[/latex] = [latex]\dfrac{cos t}{sin t}[/latex]
Example 5
Using Identities to Evaluate Trigonometric Functions
ⓐ Given [latex]sin (45°)[/latex] = [latex]\frac{\sqrt{2}}{2}[/latex], [latex]cos (45°)[/latex] = [latex]\frac{\sqrt{2}}{2}[/latex], evaluate [latex]tan (45°)[/latex].
ⓑ Given [latex]sin[/latex] ([latex]\frac{5π}{6}[/latex]) = [latex]\frac{1}{2}[/latex], [latex]cos[/latex] ([latex]\frac{5π}{6}[/latex]) = –[latex]\frac{\sqrt{3}}{2}[/latex], evaluate [latex]sec[/latex] ([latex]\frac{5π}{6}[/latex]).
Show/Hide Solution
Solution
Because we know the sine and cosine values for these angles, we can use identities to evaluate the other functions.
ⓐ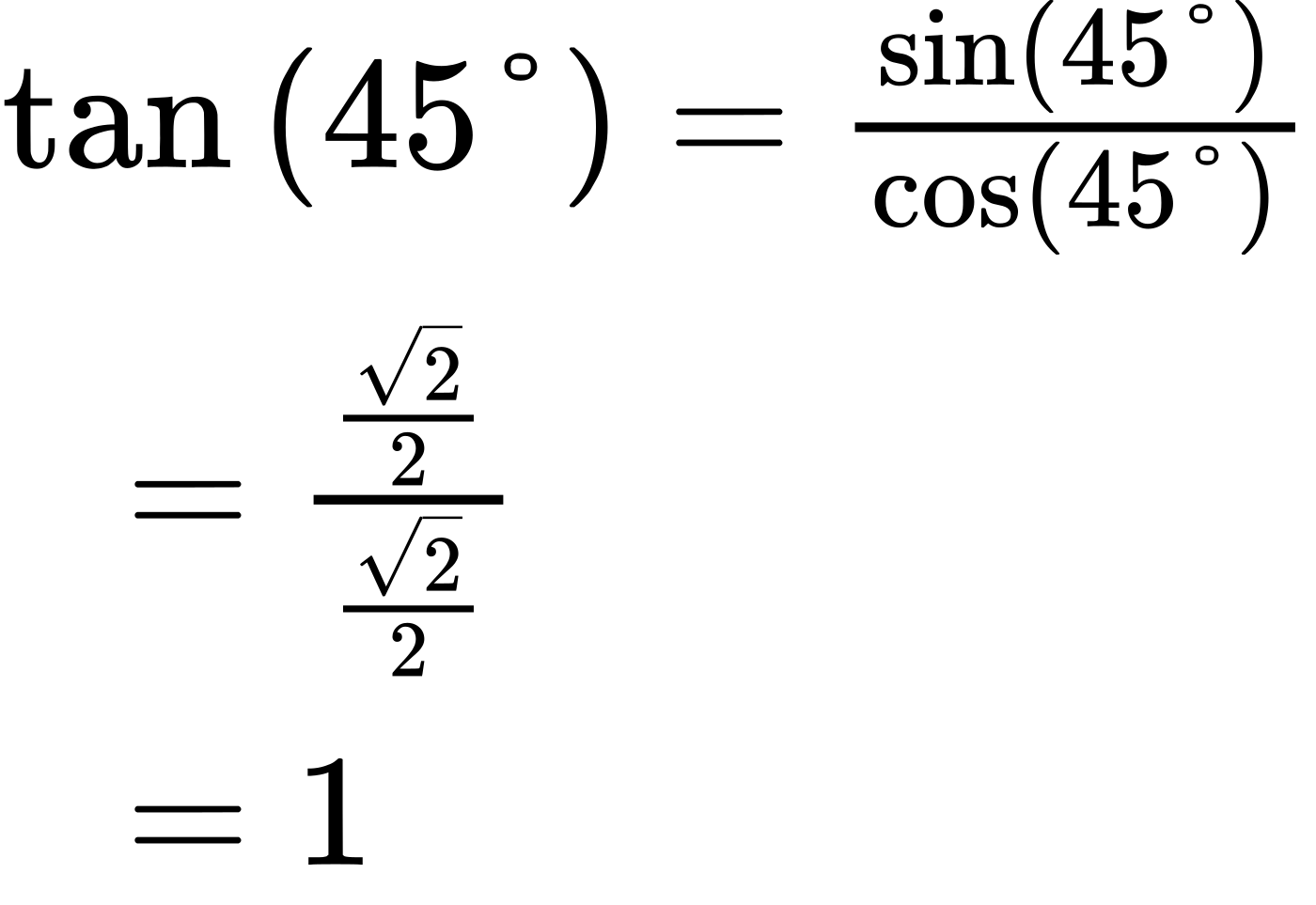
ⓑ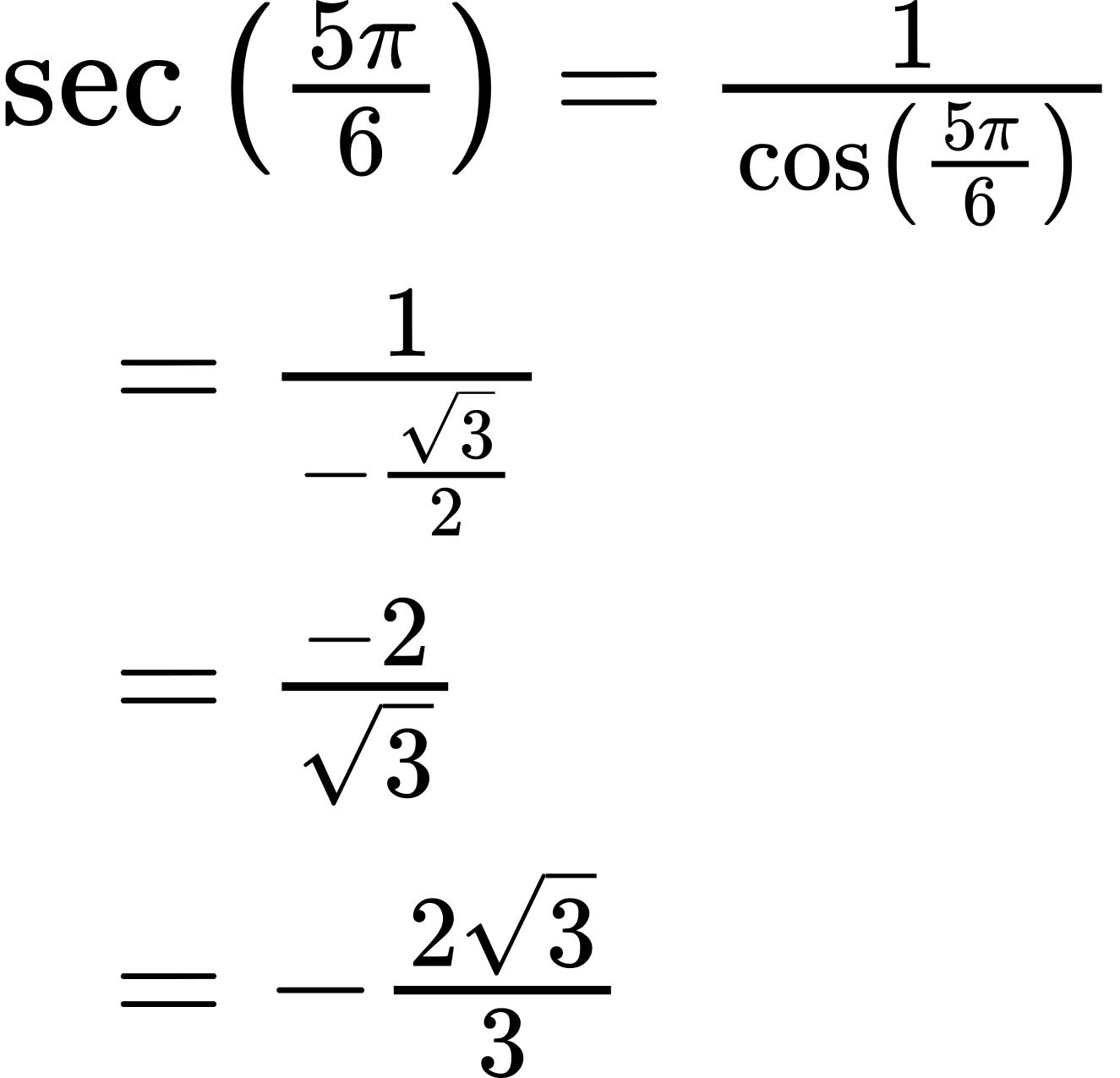
Try It #5
Evaluate [latex]csc[/latex] ([latex]\frac{7π}{6}[/latex]).
Example 6
Using Identities to Simplify Trigonometric Expressions
Simplify [latex]\frac{\text{sec t}}{\text{tan t}}[/latex].
Show/Hide Solution
Solution
We can simplify this by rewriting both functions in terms of sine and cosine.

By showing that [latex]\frac{\text{sec t}}{\text{tan t}}[/latex] can be simplified to [latex]csc t[/latex], we have, in fact, established a new identity.
Try It #6
Simplify ([latex]tan t[/latex])([latex]cos t[/latex]).
Alternate Forms of the Pythagorean Identity
We can use these fundamental identities to derive alternative forms of the Pythagorean Identity, [latex]cos^2t[/latex] + [latex]sin^2t[/latex] = [latex]1[/latex]. One form is obtained by dividing both sides by [latex]cos^2t[/latex]:
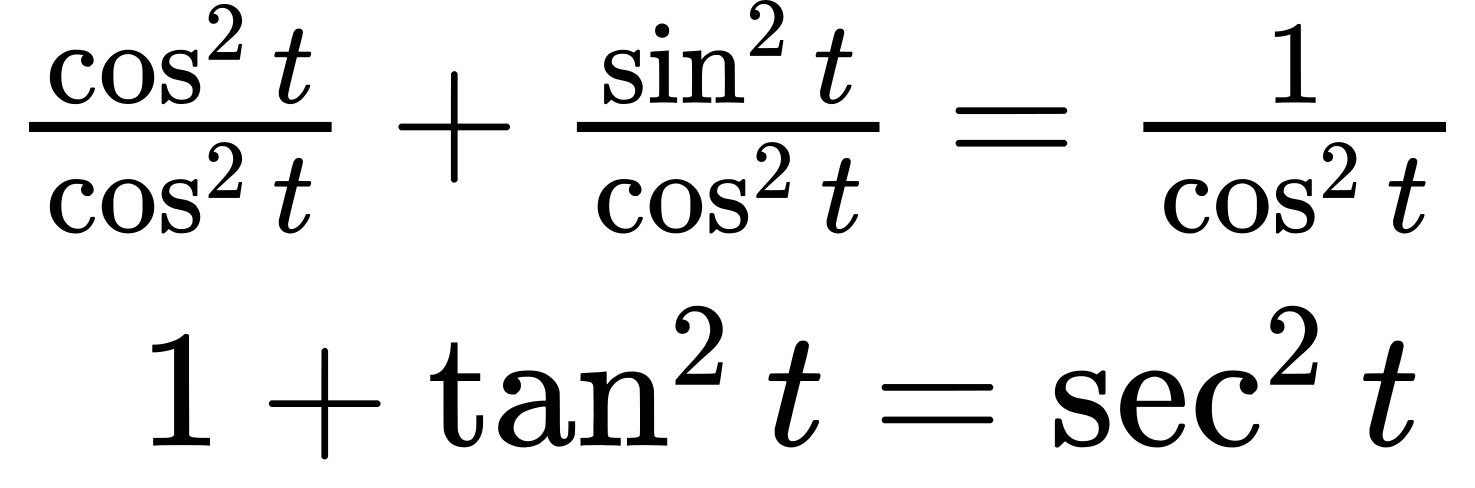
The other form is obtained by dividing both sides by [latex]sin^2t[/latex]:
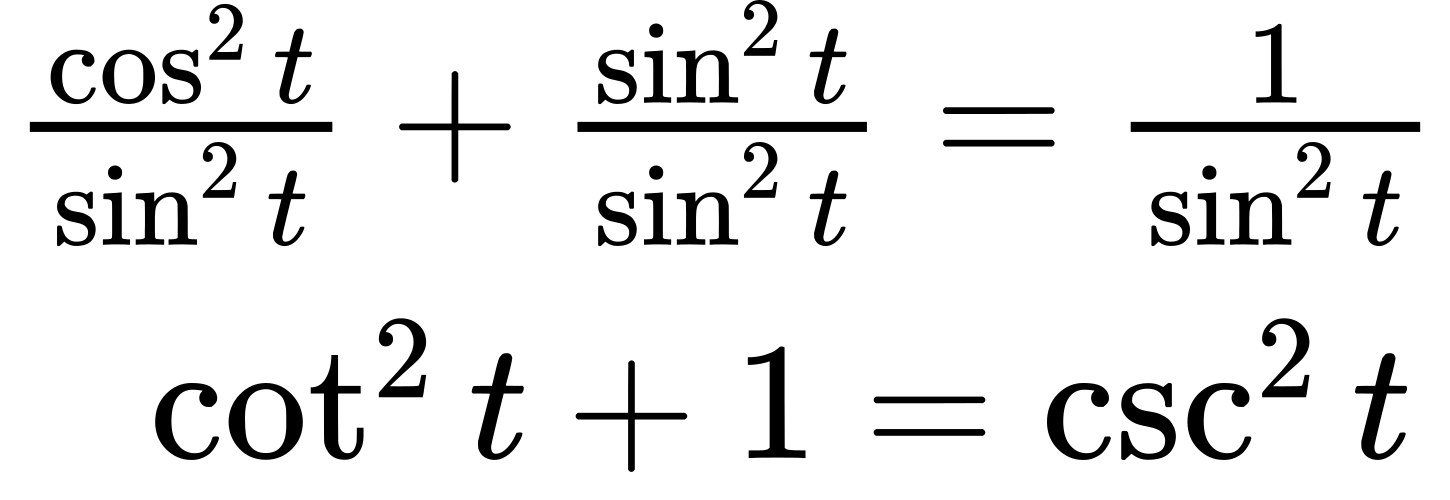
Alternate Forms of the Pythagorean Identity
Example 7
Using Identities to Relate Trigonometric Functions
If [latex]cos(t)[/latex] = [latex]\frac{12}{13}[/latex] and [latex]t[/latex] is in quadrant IV, as shown in Figure 8, find the values of the other five trigonometric functions.
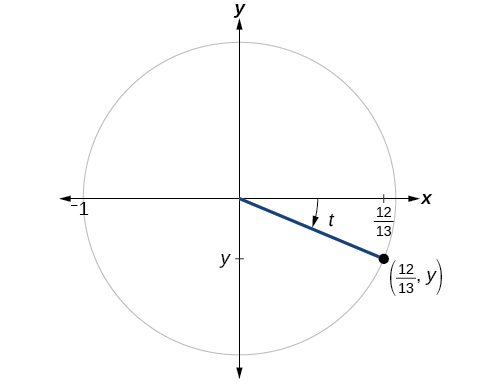
Show/Hide Solution
Solution
We can find the sine using the Pythagorean Identity, [latex]cos^2t[/latex] + [latex]sin^2t[/latex] = [latex]1[/latex], and the remaining functions by relating them to sine and cosine.

The sign of the sine depends on the y–values in the quadrant where the angle is located. Since the angle is in quadrant IV, where the y–values are negative, its sine is negative, –[latex]\frac{5}{13}[/latex].
The remaining functions can be calculated using identities relating them to sine and cosine.

Try It #7
If [latex]sec(t)[/latex] = –[latex]\frac{17}{8}[/latex] and 0 < [latex]t[/latex] < [latex]π[/latex], find the values of the other five functions.
As we discussed in the chapter opening, a function that repeats its values in regular intervals is known as a periodic function. The trigonometric functions are periodic. For the four trigonometric functions, sine, cosine, cosecant and secant, a revolution of one circle, or [latex]2π[/latex], will result in the same outputs for these functions. And for tangent and cotangent, only a half a revolution will result in the same outputs.
Other functions can also be periodic. For example, the lengths of months repeat every four years. If x represents the length time, measured in years, and [latex]f(x)[/latex] represents the number of days in February, then [latex]f(x+4)[/latex] = [latex]f(x)[/latex]. This pattern repeats over and over through time. In other words, every four years, February is guaranteed to have the same number of days as it did 4 years earlier. The positive number 4 is the smallest positive number that satisfies this condition and is called the period. A period is the shortest interval over which a function completes one full cycle—in this example, the period is 4 and represents the time it takes for us to be certain February has the same number of days.
Period of a Function
The period [latex]P[/latex] of a repeating function [latex]f[/latex] is the number representing the interval such that [latex]f(x + P)[/latex] = [latex]f(x)[/latex] for any value of [latex]x[/latex].
The period of the cosine, sine, secant, and cosecant functions is [latex]2π[/latex].
The period of the tangent and cotangent functions is [latex]π[/latex].
Example 8
Finding the Values of Trigonometric Functions
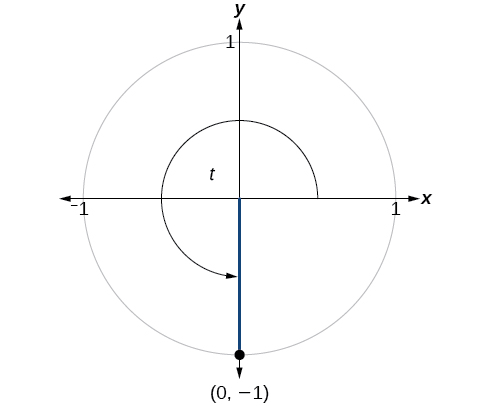
Find the values of the six trigonometric functions of angle [latex]t[/latex] based on Figure 9.
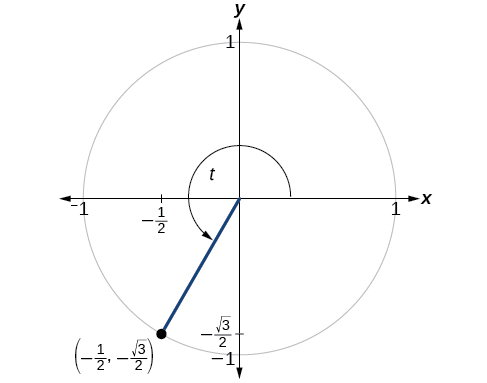
Show/Hide Solution
Solution
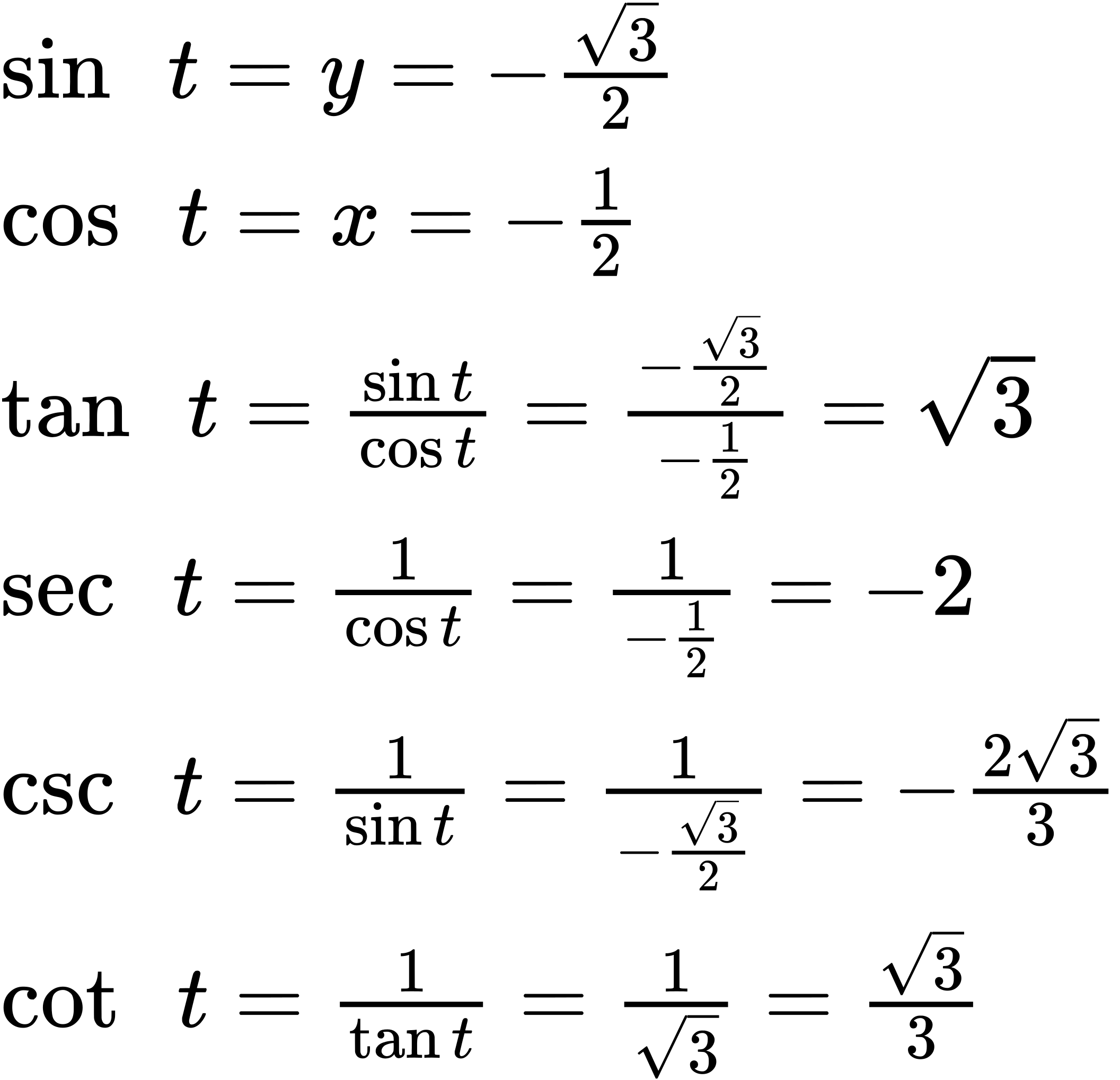
Try It #8
Find the values of the six trigonometric functions of angle [latex]t[/latex] based on Figure 10.
Example 9
Finding the Value of Trigonometric Functions
If [latex]sin(t)[/latex] = –[latex]\frac{\sqrt{3}}{2}[/latex] and [latex]cos(t)[/latex] = [latex]\frac{1}{2}[/latex], find [latex]sec(t)[/latex], [latex]csc(t)[/latex], [latex]tan(t)[/latex], [latex]cot(t)[/latex].
Show/Hide Solution
Solution
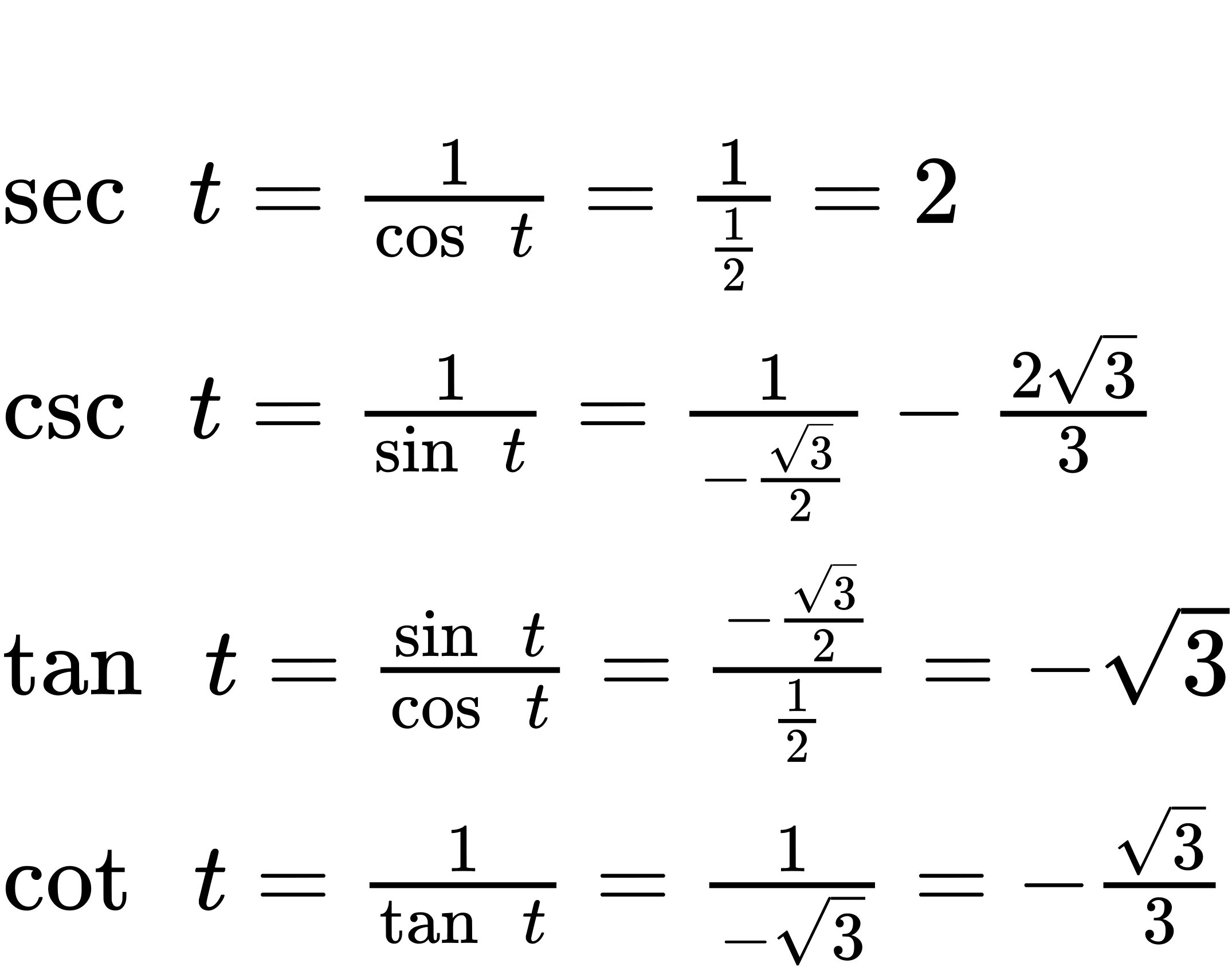
Try It #9
If [latex]sin(t)[/latex] = [latex]\frac{\sqrt{2}}{2}[/latex] and [latex]cos(t)[/latex] = [latex]\frac{\sqrt{2}}{2}[/latex], find [latex]sec(t)[/latex], [latex]csc(t)[/latex], [latex]tan(t)[/latex], and [latex]cot(t)[/latex].
Media
Access these online resources for additional instruction and practice with other trigonometric functions.
- Determining Trig Function Values
- More Examples of Determining Trig Functions
- Pythagorean Identities
- Trig Functions on a Calculator
Section Exercises
Verbal
1. On an interval of [0, [latex]2π[/latex]), can the sine and cosine values of a radian measure ever be equal? If so, where?
2. What would you estimate the cosine of [latex]π[/latex] degrees to be? Explain your reasoning.
3. For any angle in quadrant II, if you knew the sine of the angle, how could you determine the cosine of the angle?
4. Describe the secant function.
5. Tangent and cotangent have a period of π. What does this tell us about the output of these functions?
Algebraic
For the following exercises, find the exact value of each expression.
6. [latex]tan[/latex] [latex]\frac{π}{6}[/latex]
7. [latex]sec[/latex] [latex]\frac{π}{6}[/latex]
8. [latex]csc[/latex] [latex]\frac{π}{6}[/latex]
9. [latex]cot[/latex] [latex]\frac{π}{6}[/latex]
10. [latex]tan[/latex] [latex]\frac{π}{4}[/latex]
11. [latex]sec[/latex] [latex]\frac{π}{4}[/latex]
12. [latex]csc[/latex] [latex]\frac{π}{4}[/latex]
13. [latex]cot[/latex] [latex]\frac{π}{4}[/latex]
14. [latex]tan[/latex] [latex]\frac{π}{3}[/latex]
15. [latex]sec[/latex] [latex]\frac{π}{3}[/latex]
16. [latex]csc[/latex] [latex]\frac{π}{3}[/latex]
17. [latex]cot[/latex] [latex]\frac{π}{3}[/latex]
For the following exercises, use reference angles to evaluate the expression.
18. [latex]tan[/latex] [latex]\frac{5π}{6}[/latex]
19. [latex]sec[/latex] [latex]\frac{7π}{6}[/latex]
20. [latex]csc[/latex] [latex]\frac{11π}{6}[/latex]
21. [latex]cot[/latex] [latex]\frac{13π}{6}[/latex]
22. [latex]tan[/latex] [latex]\frac{7π}{4}[/latex]
23. [latex]sec[/latex] [latex]\frac{3π}{4}[/latex]
24. [latex]csc[/latex] [latex]\frac{5π}{4}[/latex]
25. [latex]cot[/latex] [latex]\frac{11π}{4}[/latex]
26. [latex]tan[/latex] [latex]\frac{8π}{3}[/latex]
27. [latex]sec[/latex] [latex]\frac{4π}{3}[/latex]
28. [latex]csc[/latex] [latex]\frac{2π}{3}[/latex]
29. [latex]cot[/latex] [latex]\frac{5π}{3}[/latex]
30. [latex]tan[/latex] 225°
31. [latex]sec[/latex] 300°
32. [latex]csc[/latex] 150°
33. [latex]cot[/latex] 240°
34. [latex]tan[/latex] 330°
35. [latex]sec[/latex] 120°
36. [latex]csc[/latex] 210°
37. [latex]cot[/latex] 315°
38. If [latex]sin t[/latex] = [latex]\frac{3}{4}[/latex], and [latex]t[/latex] is in quadrant II, find [latex]cos t[/latex], [latex]sec t[/latex], [latex]csc t[/latex], [latex]tan t[/latex], [latex]cot t[/latex].
39. If [latex]cos t[/latex] = –[latex]\frac{1}{2}[/latex], and [latex]t[/latex] is in quadrant III, find [latex]sin t[/latex], [latex]sec t[/latex], [latex]csc t[/latex], [latex]tan t[/latex], [latex]cot t[/latex].
40. If [latex]tan t[/latex] = [latex]\frac{12}{5}[/latex], and 0 ≤ [latex]t[/latex] < [latex]\frac{π}{2}[/latex], find [latex]sin t[/latex], [latex]cos t[/latex], [latex]sec t[/latex], [latex]csc t[/latex], and [latex]cot t[/latex].
41. If [latex]sin t[/latex] = [latex]\frac{\sqrt{3}}{2}[/latex] and [latex]cos t[/latex] = [latex]\frac{1}{2}[/latex], find [latex]sec t[/latex], [latex]csc t[/latex], [latex]tan t[/latex], and [latex]cot t[/latex].
42. If [latex]sin[/latex] 40° ≈ 0.643 and [latex]cos[/latex] 40° ≈ 0.766 find [latex]sec[/latex] 40°, [latex]csc[/latex] 40°, [latex]tan[/latex] 40°, and [latex]cot[/latex] 40°.
43. If [latex]sin t[/latex] = [latex]\frac{\sqrt{2}}{2}[/latex], what is the [latex]sin(−t)[/latex]?
44. If [latex]cos t[/latex] = [latex]\frac{1}{2}[/latex], what is the [latex]cos(−t)[/latex]?
45. If [latex]sec t[/latex] = 3.1, what is the [latex]sec(−t)[/latex]?
46. If [latex]csc t[/latex] = 0.34, what is the [latex]csc(−t)[/latex]?
47. If [latex]tan t[/latex] = −1.4, what is the [latex]tan(−t)[/latex]?
48. If [latex]cot t[/latex] = 9.23, what is the [latex]cot(−t)[/latex]?
Graphical
For the following exercises, use the angle in the unit circle to find the value of the each of the six trigonometric functions.
49.
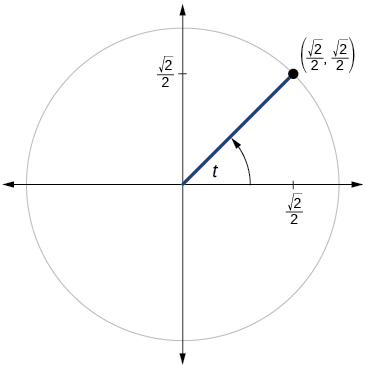
50.
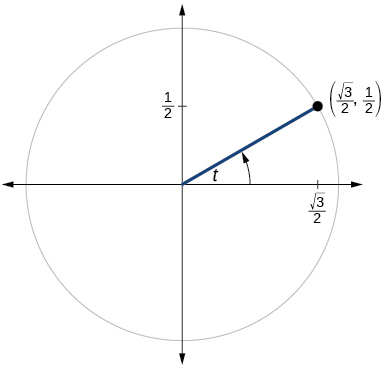
51.
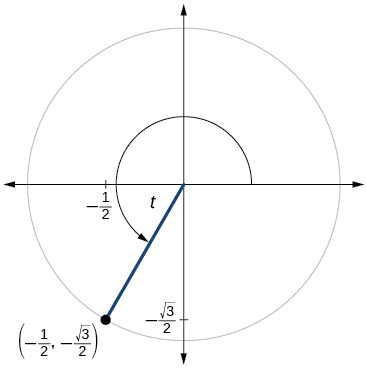
Extensions
For the following exercises, use identities to evaluate the expression.
52. If [latex]tan t[/latex] ≈ 2.7, and [latex]sin t[/latex] ≈ 0.94, find [latex]cos t[/latex].
53. If [latex]tan t[/latex] ≈ 1.3, and [latex]cos t[/latex] ≈ 0.61, find [latex]sin t[/latex].
54. If [latex]csc t[/latex] ≈ 3.2, and [latex]cos t[/latex] ≈ 0.95, find [latex]tan t[/latex].
55. If [latex]cot t[/latex] ≈ 0.58, and [latex]cos t[/latex] ≈ 0.5, find [latex]csc t[/latex].
56. Determine whether the function [latex]f(x)[/latex] = [latex]2sin[/latex] [latex]x[/latex] [latex]cos x[/latex] is even, odd, or neither.
57. Determine whether the function [latex]f(x)[/latex] = [latex]3sin^2x[/latex] [latex]cos x[/latex] + [latex]sec x[/latex] is even, odd, or neither.
58. Determine whether the function [latex]f(x)[/latex] = [latex]sin x[/latex] − [latex]2cos^2x[/latex] is even, odd, or neither.
59. Determine whether the function [latex]f(x)[/latex] = [latex]csc^2x[/latex] + [latex]sec x[/latex] is even, odd, or neither.
For the following exercises, use identities to simplify the expression.
60. [latex]csc t[/latex] [latex]tan t[/latex]
61. [latex]\frac{\text{sec t}}{\text{csc t}}[/latex]
Real-World Applications
62. The amount of sunlight in a certain city can be modeled by the function [latex]h[/latex] = [latex]15[/latex] [latex]cos[/latex] ([latex]\frac{1}{600}[/latex] [latex]d[/latex]), where [latex]h[/latex] represents the hours of sunlight, and [latex]d[/latex] is the day of the year. Use the equation to find how many hours of sunlight there are on February 11, the 42nd day of the year. State the period of the function.
63. The amount of sunlight in a certain city can be modeled by the function [latex]h[/latex] = [latex]16[/latex] [latex]cos[/latex] ([latex]\frac{1}{500}[/latex] [latex]d[/latex]), where [latex]h[/latex] represents the hours of sunlight, and [latex]d[/latex] is the day of the year. Use the equation to find how many hours of sunlight there are on September 24, the 267th day of the year. State the period of the function.
64. The equation [latex]P[/latex] = [latex]20[/latex] [latex]sin[/latex]([latex]2πt[/latex]) + [latex]100[/latex] models the blood pressure, [latex]P[/latex], where [latex]t[/latex] represents time in seconds. (a) Find the blood pressure after 15 seconds. (b) What are the maximum and minimum blood pressures?
65. The height of a piston, [latex]h[/latex], in inches, can be modeled by the equation [latex]h[/latex] = [latex]2[/latex] [latex]cos[/latex] [latex]x[/latex] + [latex]6[/latex], where [latex]x[/latex] represents the crank angle. Find the height of the piston when the crank angle is 30°.
66. The height of a piston, [latex]h[/latex], in inches, can be modeled by the equation [latex]h[/latex] = [latex]2[/latex] [latex]cos[/latex] [latex]x[/latex] + [latex]5[/latex], where [latex]x[/latex] represents the crank angle. Find the height of the piston when the crank angle is 55°.