Section 2.2 – The Unit Circle: Sine and Cosine Functions
Learning Objectives
Welcome to Section 2.2! In this section, you will…
- Find function values for the sine and cosine of
[latex]\text{30° or}[/latex] ([latex]\frac{π}{6}[/latex]), [latex]\text{45° or}[/latex] ([latex]\frac{π}{4}[/latex]), and [latex]\text{60° or}[/latex] ([latex]\frac{π}{3}[/latex]). - Identify the domain and range of sine and cosine functions.
- Use reference angles to evaluate trigonometric functions.

Looking for a thrill? Then consider a ride on the Ain Dubai, the world’s tallest Ferris wheel. Located in Dubai, the most populous city and the financial and tourism hub of the United Arab Emirates, the wheel soars to 820 feet, about 1.5 tenths of a mile. Described as an observation wheel, riders enjoy spectacular views of the Burj Khalifa (the world’s tallest building) and the Palm Jumeirah (a human-made archipelago home to over 10,000 people and 20 resorts) as they travel from the ground to the peak and down again in a repeating pattern. A ride in the Ferris wheel during the Texas Folklife Festival at the Institute of Texan Cultures gives riders a birdseye view of the fairgrounds in Hemisfair Park.

In this section, we will examine this type of revolving motion around a circle. To do so, we need to define the type of circle first, and then place that circle on a coordinate system. Then we can discuss circular motion in terms of the coordinate pairs.
Finding Function Values for the Sine and Cosine
To define our trigonometric functions, we begin by drawing a unit circle, a circle centered at the origin with radius 1, as shown in Figure 2. The angle (in radians) that [latex]t[/latex] intercepts forms an arc of length [latex]s[/latex]. Using the formula [latex]s = rt[/latex], and knowing that [latex]r = 1[/latex], we see that for a unit circle, [latex]s = t[/latex].
Recall that the x- and y-axes divide the coordinate plane into four quarters called quadrants. We label these quadrants to mimic the direction a positive angle would sweep. The four quadrants are labeled I, II, III, and IV.
For any angle [latex]t[/latex], we can label the intersection of the terminal side and the unit circle as by its coordinates, [latex](x,y)[/latex]. The coordinates [latex]x[/latex] and [latex]y[/latex] will be the outputs of the trigonometric functions [latex]f(t)[/latex] = [latex]cos t[/latex] and [latex]f(t)[/latex] = [latex]sin t[/latex], respectively. This means [latex]x[/latex] = [latex]cos t[/latex] and [latex]y[/latex] = [latex]sin t[/latex].
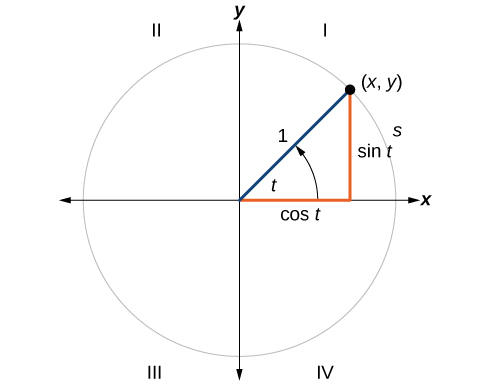
Unit Circle
A unit circle has a center at (0,0) and radius 1. In a unit circle, the length of the intercepted arc is equal to the radian measure of the central angle [latex]t[/latex]. Let [latex](x,y)[/latex] be the endpoint on the unit circle of an arc of arc length [latex]s[/latex]. The [latex](x,y)[/latex] coordinates of this point can be described as functions of the angle.
Defining Sine and Cosine Functions
Now that we have our unit circle labeled, we can learn how the [latex](x,y)[/latex] coordinates relate to the arc length and angle. The sine function relates a real number [latex]t[/latex] to the y-coordinate of the point where the corresponding angle intercepts the unit circle. More precisely, the sine of an angle t equals the y-value of the endpoint on the unit circle of an arc of length [latex]t[/latex]. In Figure 2, the sine is equal to [latex]y[/latex]. Like all functions, the sine function has an input and an output. Its input is the measure of the angle; its output is the y-coordinate of the corresponding point on the unit circle.
The cosine function of an angle [latex]t[/latex] equals the x–value of the endpoint on the unit circle of an arc of length [latex]t[/latex]. In Figure 3, the cosine is equal to [latex]x[/latex].

Because it is understood that sine and cosine are functions, we do not always need to write them with parentheses: [latex]sin t[/latex] is the same as [latex]sin(t)[/latex] and [latex]cos t[/latex] is the same as [latex]cos(t)[/latex]. Likewise, [latex]cos^2t[/latex] is a commonly used shorthand notation for [latex](cos(t))^2.[/latex] Be aware that many calculators and computers do not recognize the shorthand notation. When in doubt, use the extra parentheses when entering calculations into a calculator or computer.
Sine and Cosine Functions
If [latex]t[/latex] is a real number and a point [latex](x,y)[/latex] on the unit circle corresponds to an angle of [latex]t[/latex], then
How To
Given a point [latex]P[/latex] [latex](x,y)[/latex] on the unit circle corresponding to an angle of [latex]t[/latex], find the sine and cosine.
- The sine of [latex]t[/latex] is equal to the y-coordinate of point [latex]P[/latex]: [latex]sin t[/latex] = [latex]y[/latex].
- The cosine of [latex]t[/latex] is equal to the x-coordinate of point [latex]P[/latex]: [latex]cos t[/latex] = [latex]x[/latex].
Example 1
Finding Function Values for Sine and Cosine
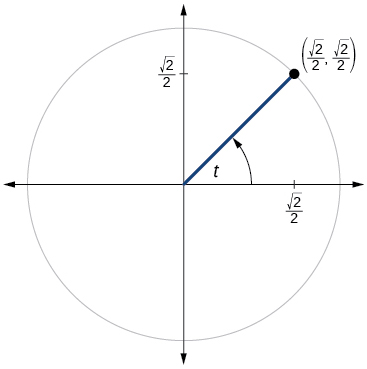
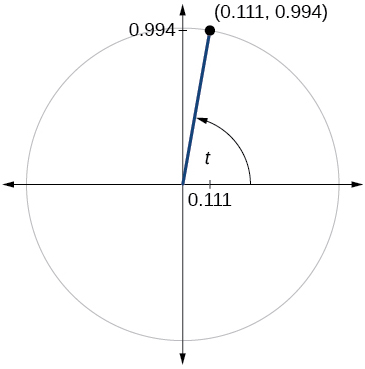
Point P is a point on the unit circle corresponding to an angle of [latex]t[/latex], as shown in Figure 4. Find [latex]cos(t)[/latex] and [latex]sin (t)[/latex].

Show/Hide Solution
Solution
We know that [latex]cos t[/latex] is the x-coordinate of the corresponding point on the unit circle and [latex]sin t[/latex] is the y-coordinate of the corresponding point on the unit circle. So:
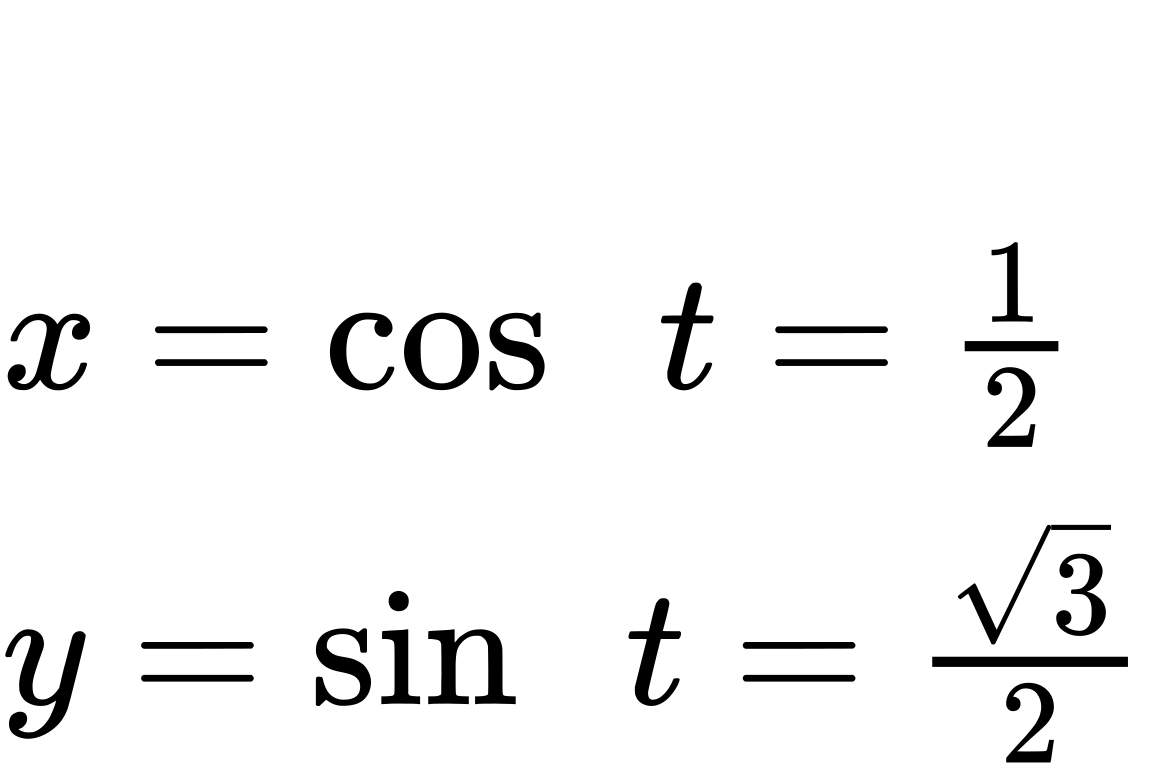
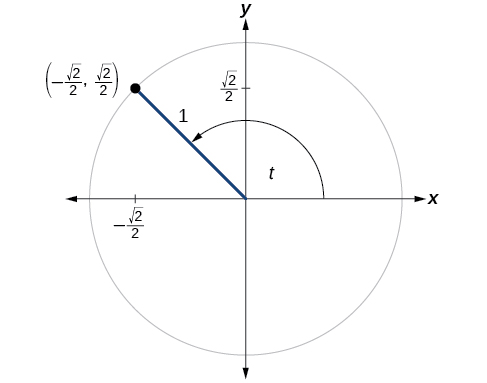
Try It #1
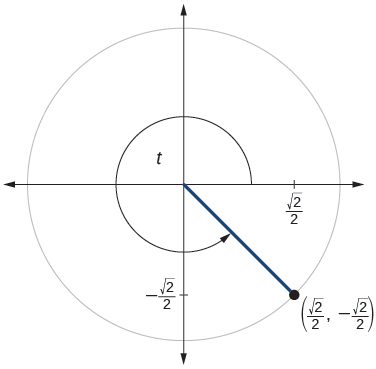
A certain angle [latex]t[/latex] corresponds to a point on the unit circle at (-[latex]\frac{\sqrt{2}}{2}[/latex], [latex]\frac{\sqrt{2}}{2}[/latex]) as shown in Figure 5. Find [latex]cos t[/latex] and [latex]sin t[/latex].
Finding Sines and Cosines of Angles on an Axis
For quadrantral angles, the corresponding point on the unit circle falls on the x- or y-axis. In that case, we can easily calculate cosine and sine from the values of [latex]x[/latex] and [latex]y[/latex].
Example 2
Calculating Sines and Cosines along an Axis
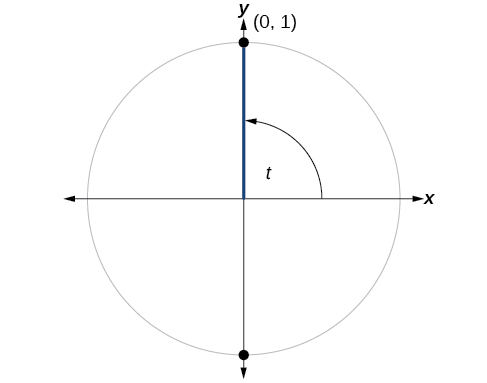
Find [latex]cos(90°)[/latex] and [latex]sin(90°)[/latex].
Show/Hide Solution
Solution
Moving 90° counterclockwise around the unit circle from the positive x-axis brings us to the top of the circle, where the [latex](x,y)[/latex] coordinates are (0, 1), as shown in Figure 6.
Using our definitions of cosine and sine,
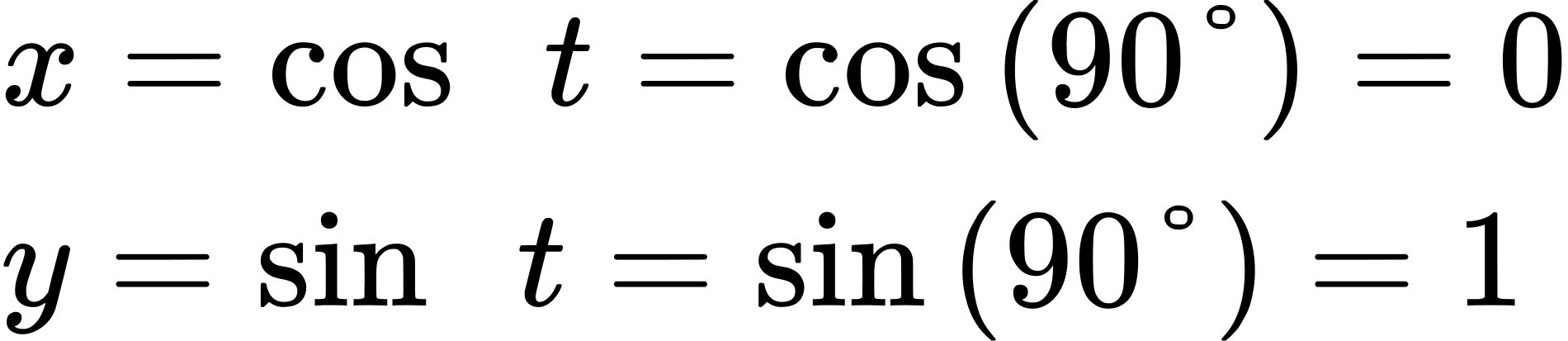
The cosine of 90° is 0; the sine of 90° is 1.
Try It #2
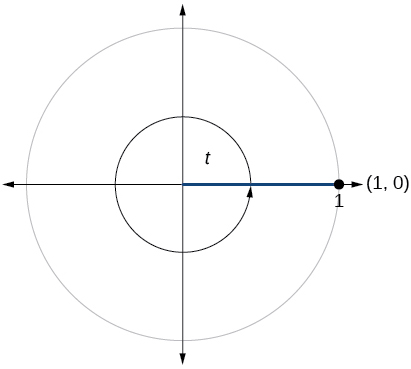
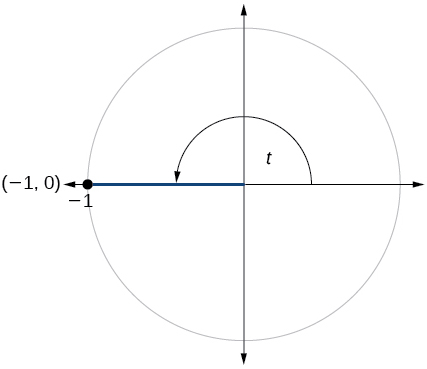
Find cosine and sine of the angle [latex]π[/latex].
The Pythagorean Identity
Now that we can define sine and cosine, we will learn how they relate to each other and the unit circle. Recall that the equation for the unit circle is [latex]x^2[/latex] + [latex]y^2[/latex] = [latex]1[/latex]. Because [latex]x[/latex] = [latex]cos t[/latex] and [latex]y[/latex] = [latex]sin t[/latex], we can substitute for [latex]x[/latex] and [latex]y[/latex] to get [latex]cos^2t[/latex] + [latex]sin^2t[/latex] = 1. This equation, [latex]cos^2t[/latex] + [latex]sin^2t[/latex] = [latex]1[/latex], is known as the Pythagorean Identity. See Figure 7.
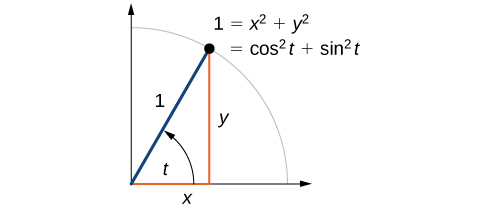
We can use the Pythagorean Identity to find the cosine of an angle if we know the sine, or vice versa. However, because the equation yields two solutions, we need additional knowledge of the angle to choose the solution with the correct sign. If we know the quadrant where the angle is, we can easily choose the correct solution.
Pythagorean Identity
The Pythagorean Identity states that, for any real number [latex]t[/latex],
How To
Given the sine of some angle [latex]t[/latex] and its quadrant location, find the cosine of [latex]t[/latex].
- Substitute the known value of [latex]sin(t)[/latex] into the Pythagorean Identity.
- Solve for [latex]cos(t)[/latex].
- Choose the solution with the appropriate sign for the x-values in the quadrant where [latex]t[/latex] is located.
Example 3
Finding a Cosine from a Sine or a Sine from a Cosine
If [latex]sin(t)[/latex] = [latex]\frac{3}{7}[/latex] and [latex]t[/latex] is in the second quadrant, find [latex]cos(t)[/latex].
Show/Hide Solution
Solution
If we drop a vertical line from the point on the unit circle corresponding to [latex]t[/latex], we create a right triangle, from which we can see that the Pythagorean Identity is simply one case of the Pythagorean Theorem. See Figure 8.

Substituting the known value for sine into the Pythagorean Identity,
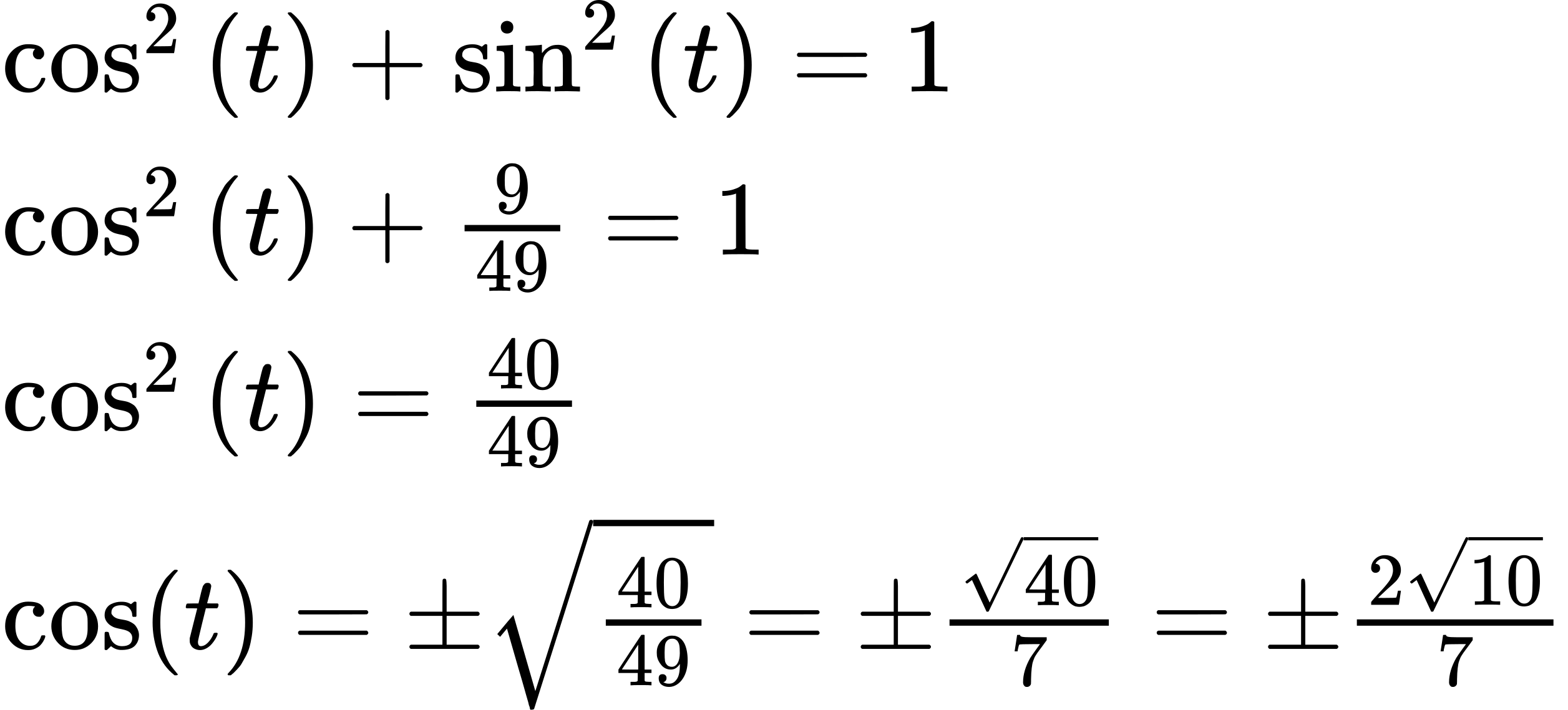
Because the angle is in the second quadrant, we know the x-value is a negative real number, so the cosine is also negative. So
Try It #3
If [latex]cos(t)[/latex] = [latex]\frac{24}{25}[/latex] and [latex]t[/latex] is in the fourth quadrant, find [latex]sin(t)[/latex].
Finding Sines and Cosines of Special Angles
We have already learned some properties of the special angles, such as the conversion from radians to degrees. We can also calculate sines and cosines of the special angles using the Pythagorean Identity and our knowledge of triangles.
Finding Sines and Cosines of 45° Angles
First, we will look at angles of 45° or [latex]\frac{π}{4}[/latex], as shown in Figure 9 . A 45°–45°–90° triangle is an isosceles triangle, so the x- and y-coordinates of the corresponding point on the circle are the same. Because the x- and y-values are the same, the sine and cosine values will also be equal.
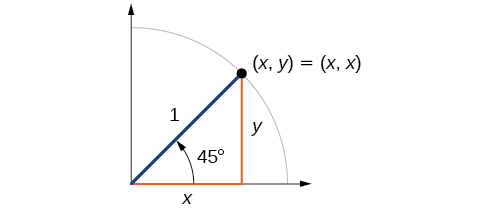
At [latex]t[/latex] = [latex]\frac{π}{4}[/latex], which is 45 degrees, the radius of the unit circle bisects the first quadrantal angle. This means the radius lies along the line [latex]y[/latex] = [latex]x[/latex]. A unit circle has a radius equal to 1. So, the right triangle formed below the line [latex]y[/latex] = [latex]x[/latex] has sides [latex]x[/latex] and [latex]y[/latex] ([latex]y = x[/latex]), and a radius = 1. See Figure 10.
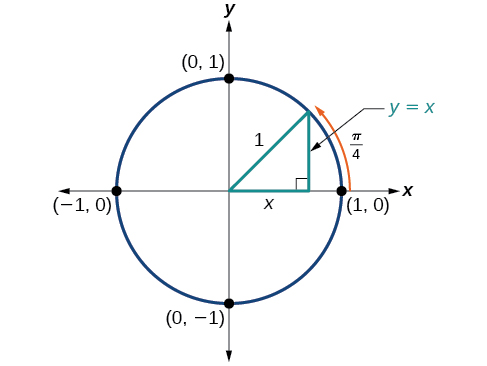
From the Pythagorean Theorem we get
Substituting [latex]y[/latex] = [latex]x[/latex], we get
Combining like terms we get
And solving for x, we get

In quadrant I, [latex]x[/latex] = [latex]\frac{1}{\sqrt{2}}[/latex].
At [latex]t[/latex] = [latex]\frac{π}{4}[/latex] or 45 degrees,
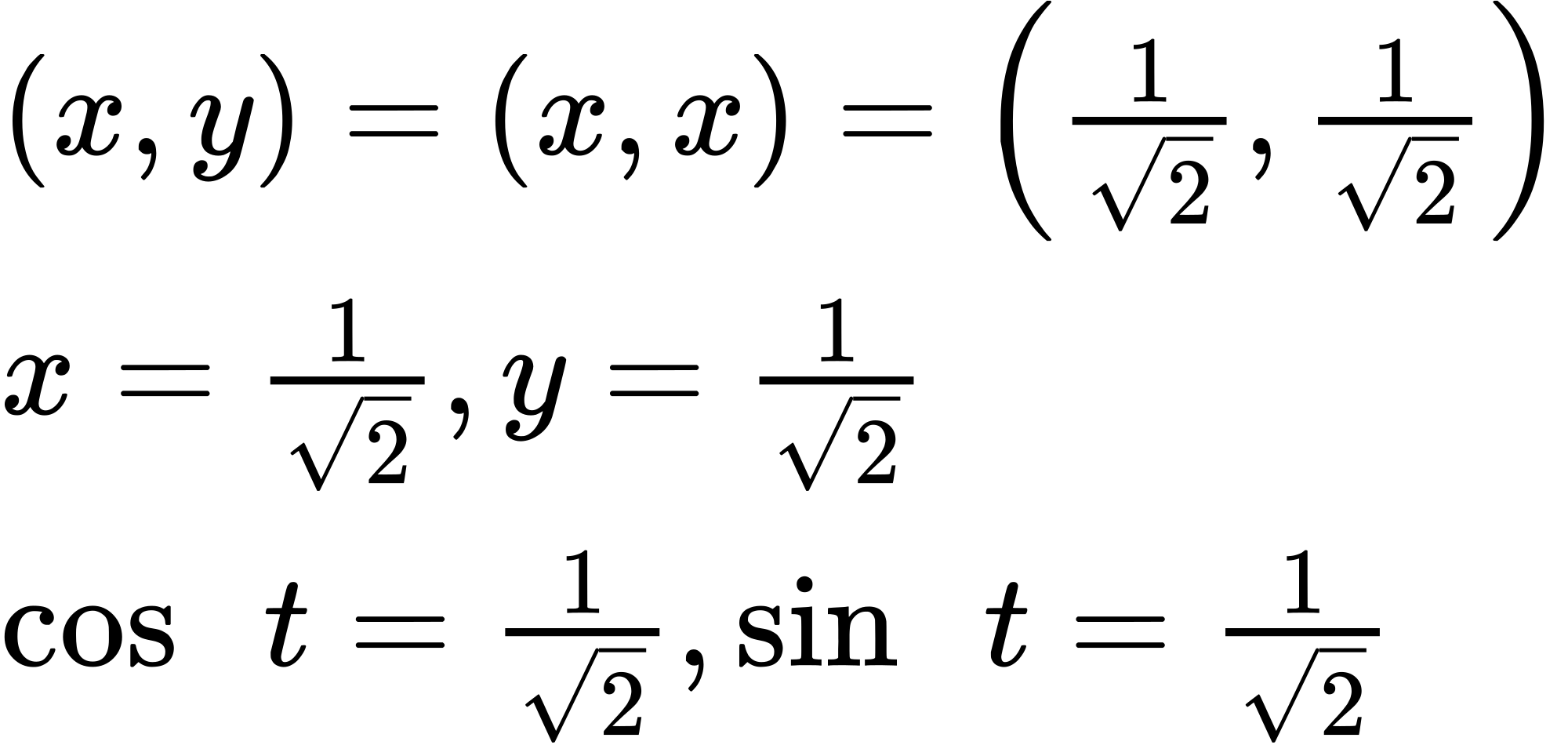
If we then rationalize the denominators, we get
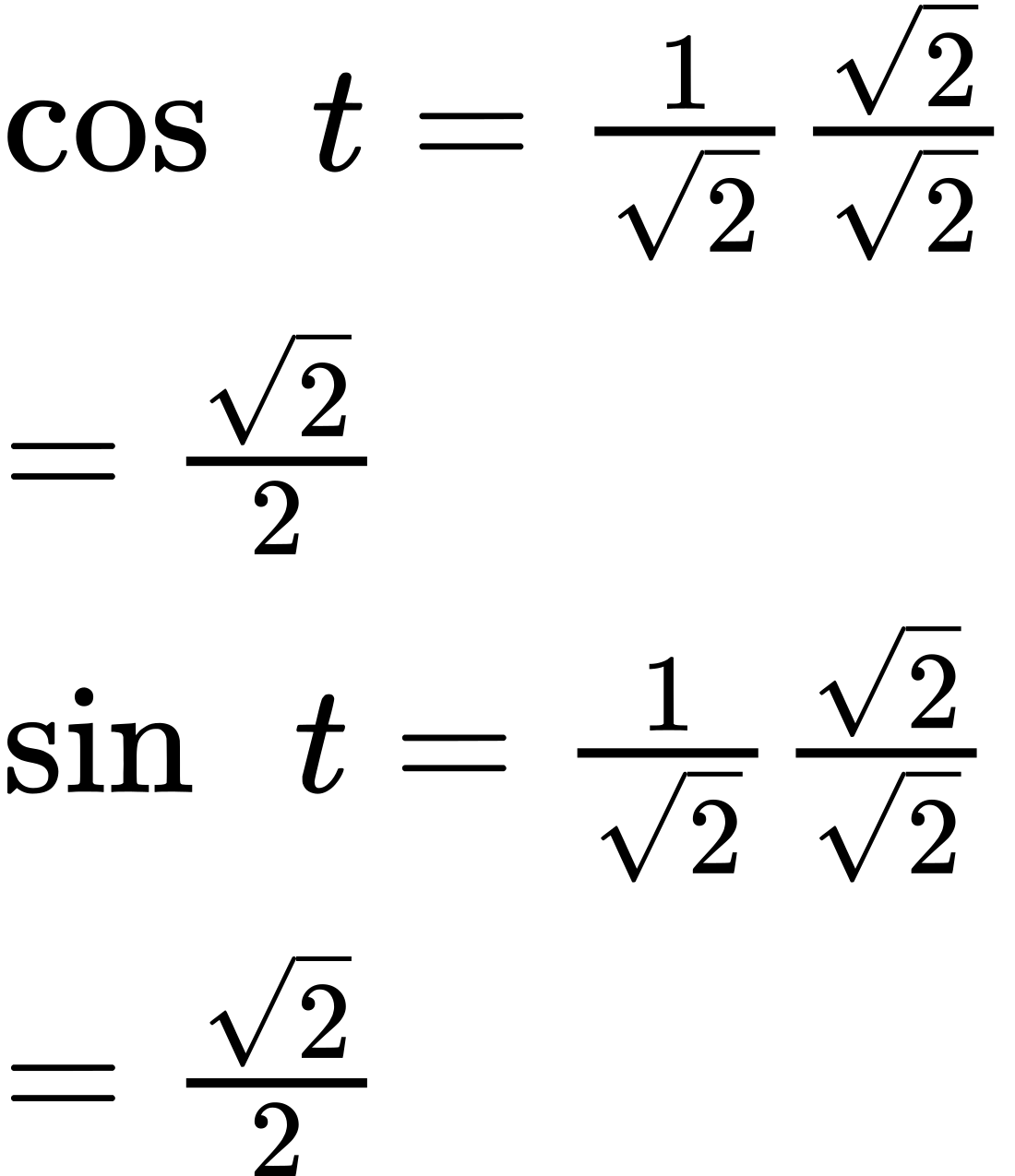
Therefore, the [latex](x,y)[/latex] coordinates of a point on a circle of radius 1 at an angle of 45° are ([latex]\frac{\sqrt{2}}{2}[/latex], [latex]\frac{\sqrt{2}}{2}[/latex]).
Finding Sines and Cosines of 30° and 60° Angles
Next, we will find the cosine and sine at an angle of 30°, or [latex]\frac{π}{6}[/latex]. First, we will draw a triangle inside a circle with one side at an angle of 30°, and another at an angle of −30°, as shown in Figure 11. If the resulting two right triangles are combined into one large triangle, notice that all three angles of this larger triangle will be 60°, as shown in Figure 12.
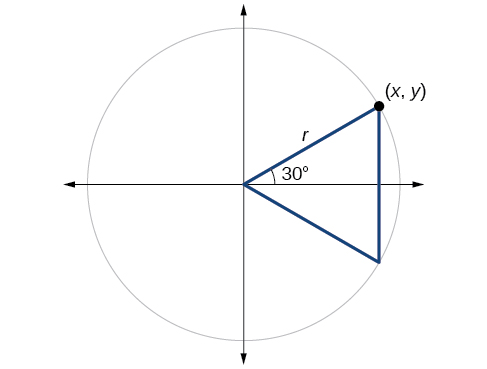

Because all the angles are equal, the sides are also equal. The vertical line has length [latex]2y[/latex], and since the sides are all equal, we can also conclude that [latex]r[/latex] = [latex]2y[/latex] or [latex]y[/latex] = [latex]\frac{1}{2}[/latex] [latex]r[/latex]. Since [latex]sin t[/latex] = [latex]y[/latex],
And since [latex]r[/latex] = 1 in our unit circle,
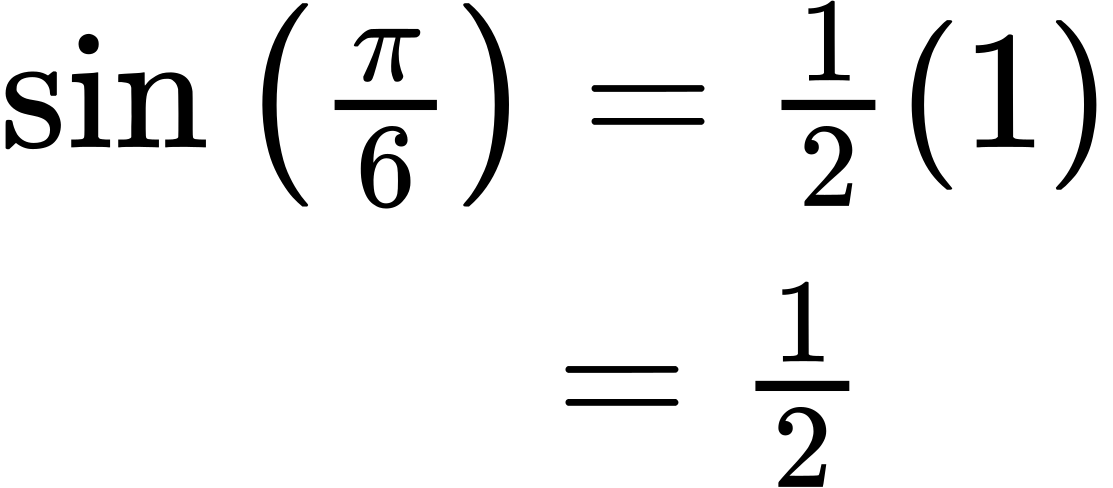
Using the Pythagorean Identity, we can find the cosine value.
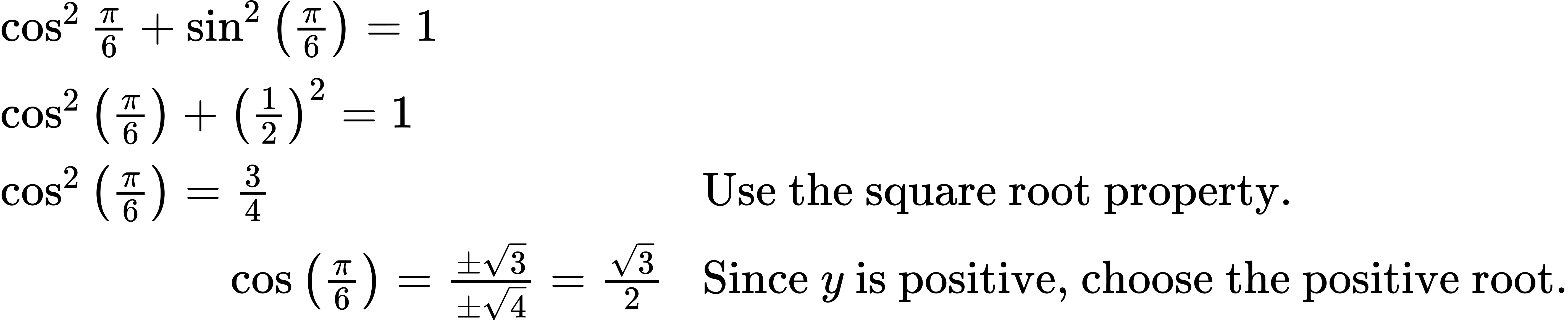
The [latex](x,y)[/latex] coordinates for the point on a circle of radius 1 at an angle of 30° are ([latex]\frac{\sqrt{3}}{2}[/latex], [latex]\frac{1}{2}[/latex]). At [latex]t[/latex] = [latex]\frac{π}{3}[/latex](60°), the radius of the unit circle, 1, serves as the hypotenuse of a 30-60-90 degree right triangle, [latex]BAD[/latex], as shown in Figure 13. Angle [latex]A[/latex] has measure 60°. At point [latex]B[/latex], we draw an angle [latex]ABC[/latex] with measure of 60°. We know the angles in a triangle sum to 180°, so the measure of angle [latex]C[/latex] is also 60°. Now we have an equilateral triangle. Because each side of the equilateral triangle [latex]ABC[/latex] is the same length, and we know one side is the radius of the unit circle, all sides must be of length 1.
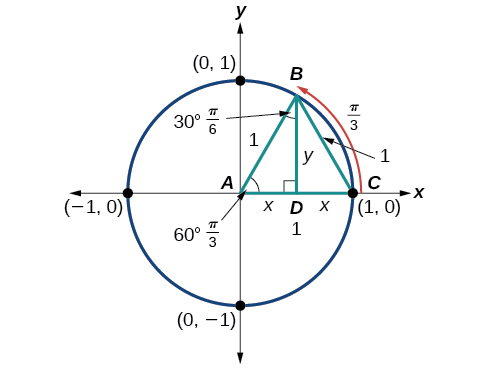
The measure of angle [latex]ABD[/latex] is 30°. So, if double, angle [latex]ABC[/latex] is 60°. [latex]BD[/latex] is the perpendicular bisector of [latex]AC[/latex], so it cuts [latex]AC[/latex] in half. This means that [latex]AD[/latex] is [latex]\frac{1}{2}[/latex] the radius, or [latex]\frac{1}{2}[/latex]. Notice that [latex]AD[/latex] is the x-coordinate of point [latex]B[/latex], which is at the intersection of the 60° angle and the unit circle. This gives us a triangle [latex]BAD[/latex] with hypotenuse of 1 and side [latex]x[/latex] of length [latex]\frac{1}{2}[/latex].
From the Pythagorean Theorem, we get
Substituting [latex]x[/latex] = [latex]\frac{1}{2}[/latex], we get
Solving for [latex]y[/latex], we get

Since [latex]t[/latex] = [latex]\frac{π}{3}[/latex] has the terminal side in quadrant I where the y-coordinate is positive, we choose [latex]y[/latex] = [latex]\frac{\sqrt{3}}{2}[/latex], the positive value.
At [latex]t[/latex] = [latex]\frac{π}{3}[/latex](60°), the [latex](x,y)[/latex] coordinates for the point on a circle of radius 1 at an angle of 60° are ([latex]\frac{1}{2}[/latex], [latex]\frac{\sqrt{3}}{2}[/latex]), so we can find the sine and cosine.
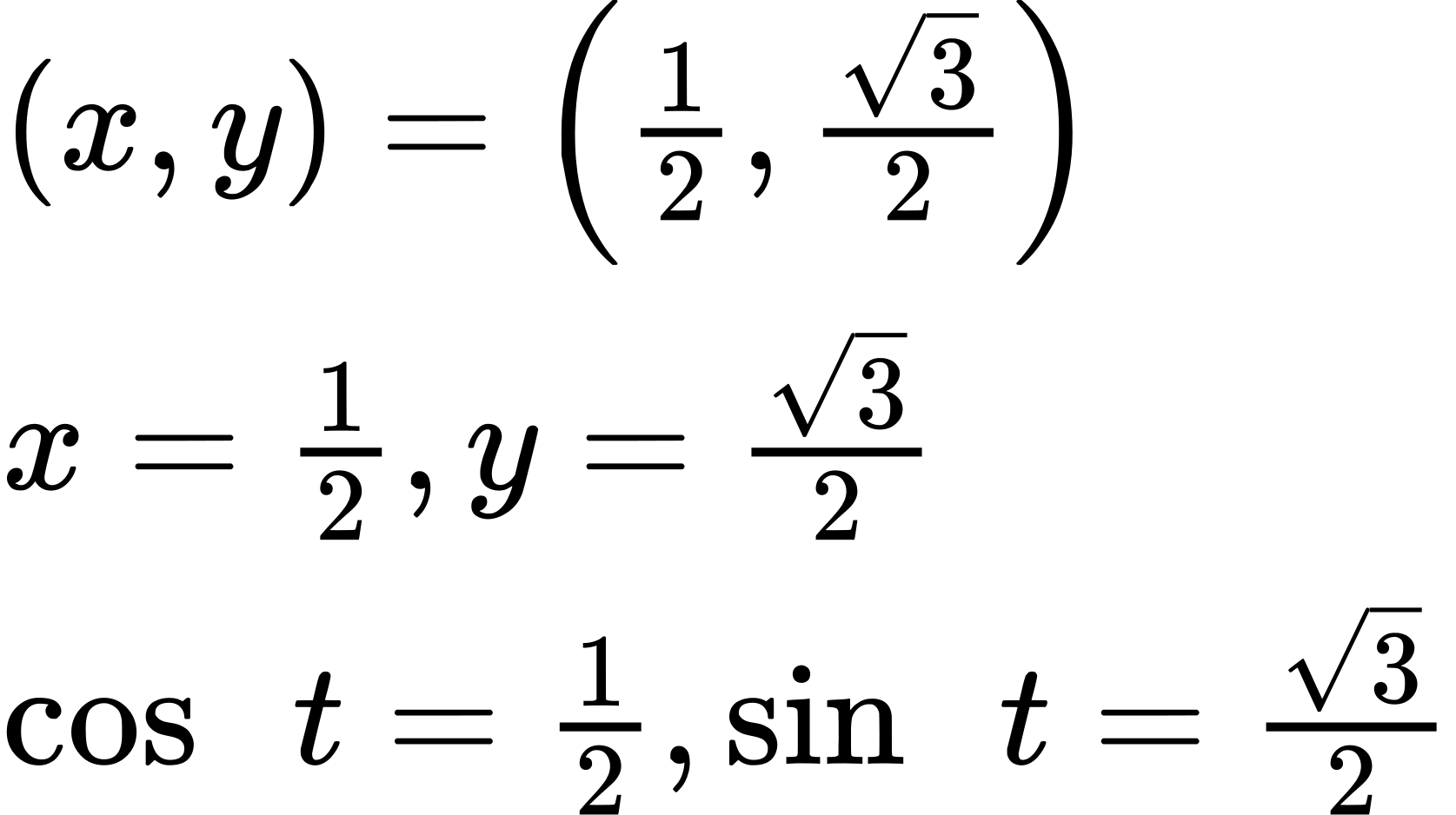
We have now found the cosine and sine values for all of the most commonly encountered angles in the first quadrant of the unit circle. Table 1 summarizes these values.
|
Angle |
0 |
[latex]\frac{π}{6}[/latex], or 30°
|
[latex]\frac{π}{4}[/latex], or 45°
|
[latex]\frac{π}{3}[/latex], or 60°
|
[latex]\frac{π}{2}[/latex], or 90°
|
|
Cosine |
1 |
[latex]\frac{\sqrt{3}}{2}[/latex] | [latex]\frac{\sqrt{2}}{2}[/latex] | [latex]\frac{1}{2}[/latex] |
0 |
|
Sine |
0 |
[latex]\frac{1}{2}[/latex] | [latex]\frac{\sqrt{2}}{2}[/latex] | [latex]\frac{\sqrt{3}}{2}[/latex] |
1 |
Figure 14 shows the common angles in the first quadrant of the unit circle.
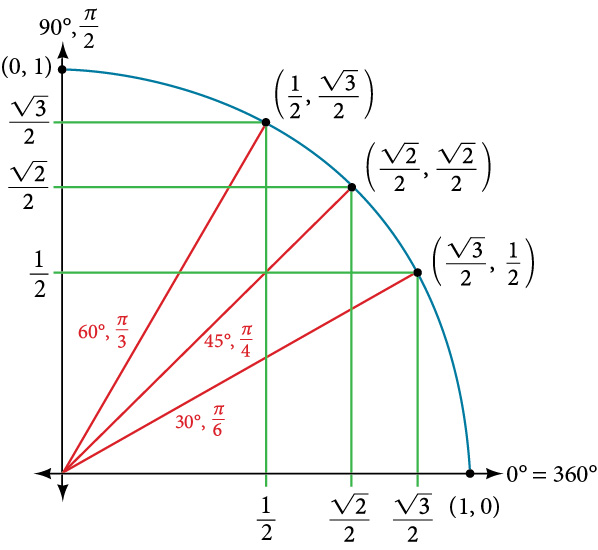
Identifying the Domain and Range of Sine and Cosine Functions
Now that we can find the sine and cosine of an angle, we need to discuss their domains and ranges. What are the domains of the sine and cosine functions? That is, what are the smallest and largest numbers that can be inputs of the functions? Because angles smaller than 0 and angles larger than [latex]2π[/latex] can still be graphed on the unit circle and have real values of [latex]x[/latex], [latex]y[/latex], and [latex]r[/latex], there is no lower or upper limit to the angles that can be inputs to the sine and cosine functions. The input to the sine and cosine functions is the rotation from the positive x-axis, and that may be any real number.
What are the ranges of the sine and cosine functions? What are the least and greatest possible values for their output? We can see the answers by examining the unit circle, as shown in Figure 15. The bounds of the x-coordinate are [−1,1]. The bounds of the y-coordinate are also [−1,1]. Therefore, the range of both the sine and cosine functions is [−1,1].

Finding Reference Angles
We have discussed finding the sine and cosine for angles in the first quadrant, but what if our angle is in another quadrant? For any given angle in the first quadrant, there is an angle in the second quadrant with the same sine value. Because the sine value is the y-coordinate on the unit circle, the other angle with the same sine will share the same y-value, but have the opposite x-value. Therefore, its cosine value will be the opposite of the first angle’s cosine value.
Likewise, there will be an angle in the fourth quadrant with the same cosine as the original angle. The angle with the same cosine will share the same x-value but will have the opposite y-value. Therefore, its sine value will be the opposite of the original angle’s sine value.
As shown in Figure 16, angle [latex]α[/latex] has the same sine value as angle [latex]t[/latex]; the cosine values are opposites. Angle [latex]β[/latex] has the same cosine value as angle [latex]t[/latex]; the sine values are opposites.
![]()

Recall that an angle’s reference angle is the acute angle, [latex]t[/latex], formed by the terminal side of the angle [latex]t[/latex] and the horizontal axis. A reference angle is always an angle between 0 and 90°, or 0 and [latex]\frac{π}{2}[/latex] radians. As we can see from Figure 17, for any angle in quadrants II, III, or IV, there is a reference angle in quadrant I.
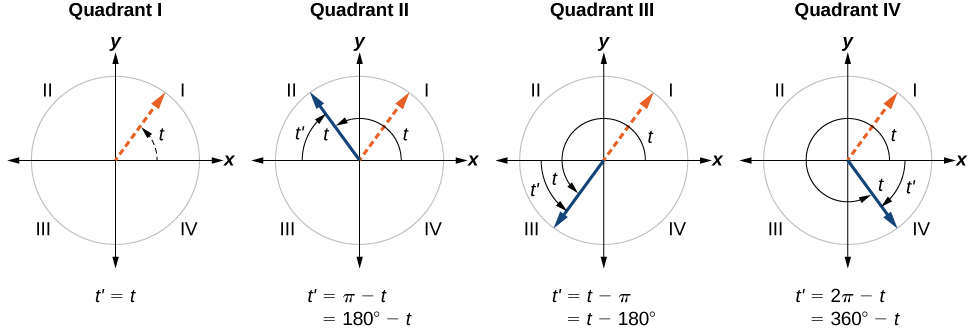
How To
Given an angle between 0 and [latex]2π[/latex], find its reference angle.
- An angle in the first quadrant is its own reference angle.
- For an angle in the second or third quadrant, the reference angle is |[latex]π[/latex]−[latex]t[/latex]| or |180°−[latex]t[/latex]|.
- For an angle in the fourth quadrant, the reference angle is [latex]2π[/latex] − [latex]t[/latex] or 360° − [latex]t[/latex].
- If an angle is less than 0 or greater than [latex]2π[/latex], add or subtract [latex]2π[/latex] as many times as needed to find an equivalent angle between 0 and [latex]2π[/latex].

Show/Hide Solution
Solution
Because 225° is in the third quadrant, the reference angle is
Try It #4
Find the reference angle of [latex]\frac{5π}{3}[/latex].
Using Reference Angles
Now let’s take a moment to reconsider the Ferris wheel introduced at the beginning of this section. Suppose a rider snaps a photograph while stopped twenty feet above ground level. The rider then rotates three-quarters of the way around the circle. What is the rider’s new elevation? To answer questions such as this one, we need to evaluate the sine or cosine functions at angles that are greater than 90 degrees or at a negative angle. Reference angles make it possible to evaluate trigonometric functions for angles outside the first quadrant. They can also be used to find [latex](x,y)[/latex] coordinates for those angles. We will use the reference angle of the angle of rotation combined with the quadrant in which the terminal side of the angle lies.
Using Reference Angles to Evaluate Trigonometric Functions
We can find the cosine and sine of any angle in any quadrant if we know the cosine or sine of its reference angle. The absolute values of the cosine and sine of an angle are the same as those of the reference angle. The sign depends on the quadrant of the original angle. The cosine will be positive or negative depending on the sign of the x-values in that quadrant. The sine will be positive or negative depending on the sign of the y-values in that quadrant.
Using Reference Angles to Find Cosine and Sine
Angles have cosines and sines with the same absolute value as cosines and sines of their reference angles. The sign (positive or negative) can be determined from the quadrant of the angle.
How To
Given an angle in standard position, find the reference angle, and the cosine and sine of the original angle.
- Measure the angle between the terminal side of the given angle and the horizontal axis. That is the reference angle.
- Determine the values of the cosine and sine of the reference angle.
- Give the cosine the same sign as the x-values in the quadrant of the original angle.
- Give the sine the same sign as the y–values in the quadrant of the original angle.
Example 5
Using Reference Angles to Find Sine and Cosine
ⓐ Using a reference angle, find the exact value of [latex]cos(150°)[/latex] and [latex]sin(150°)[/latex].
ⓑ Using the reference angle, find cos [latex]\frac{5π}{4}[/latex] and sin [latex]\frac{5π}{4}[/latex].
Show/Hide Solution
Solution
ⓐ 150° is located in the second quadrant. The angle it makes with the x-axis is 180° − 150° = 30°, so the reference angle is 30°.
This tells us that 150° has the same sine and cosine values as 30°, except for the sign. We know that
Since 150° is in the second quadrant, the x–coordinate of the point on the circle is negative, so the cosine value is negative. The y–coordinate is positive, so the sine value is positive.
ⓑ [latex]\frac{5π}{4}[/latex] is in the third quadrant. Its reference angle is [latex]\frac{5π}{4}[/latex] − [latex]π[/latex] = [latex]\frac{π}{4}[/latex]. The cosine and sine of [latex]\frac{π}{4}[/latex] are both [latex]\frac{\sqrt{2}}{2}[/latex]. In the third quadrant, both [latex]x[/latex] and [latex]y[/latex] are negative, so:
Try It #5
ⓐ Use the reference angle of 315° to find [latex]cos(315°)[/latex] and [latex]sin(315°)[/latex].
ⓑ Use the reference angle of –[latex]\frac{π}{6}[/latex] to find [latex]cos[/latex] (-[latex]\frac{π}{6}[/latex]) and [latex]sin[/latex] (-[latex]\frac{π}{6}[/latex]).
Using Reference Angles to Find Coordinates
Now that we have learned how to find the cosine and sine values for special angles in the first quadrant, we can use symmetry and reference angles to fill in cosine and sine values for the rest of the special angles on the unit circle. They are shown in Figure 19. Take time to learn the [latex](x,y)[/latex] coordinates of all of the major angles in the first quadrant.
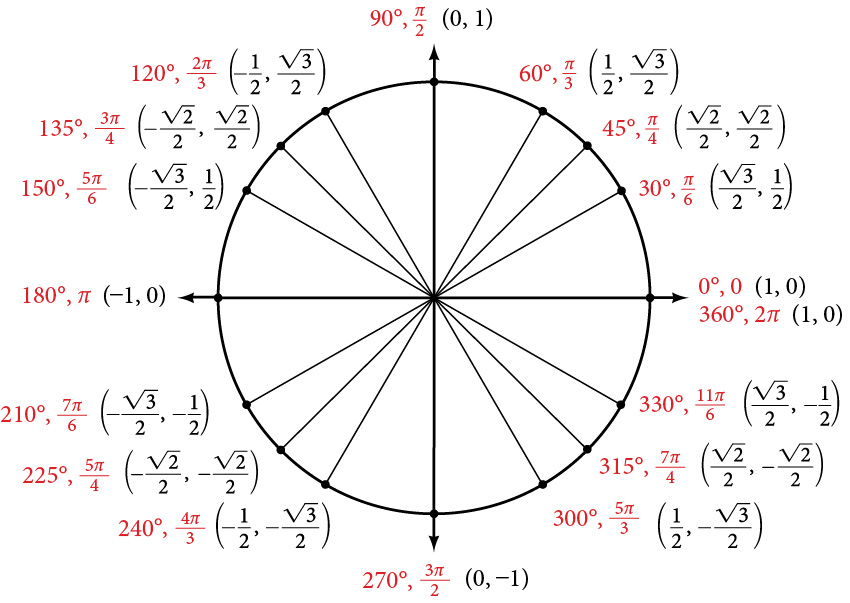
In addition to learning the values for special angles, we can use reference angles to find [latex](x,y)[/latex] coordinates of any point on the unit circle, using what we know of reference angles along with the identities
![]()
First, we find the reference angle corresponding to the given angle. Then we take the sine and cosine values of the reference angle and give them the signs corresponding to the y– and x–values of the quadrant.
How To
Given the angle of a point on a circle and the radius of the circle, find the [latex](x,y)[/latex] coordinates of the point.
- Find the reference angle by measuring the smallest angle to the x–axis.
- Find the cosine and sine of the reference angle.
- Determine the appropriate signs for [latex]x[/latex] and [latex]y[/latex] in the given quadrant.
Example 6
Using the Unit Circle to Find Coordinates
Find the coordinates of the point on the unit circle at an angle of [latex]\frac{7π}{6}[/latex].
Show/Hide Solution
Solution
We know that the angle [latex]\frac{7π}{6}[/latex] is in the third quadrant.
First, let’s find the reference angle by measuring the angle to the x–axis. To find the reference angle of an angle whose terminal side is in quadrant III, we find the difference of the angle and [latex]π[/latex].
Next, we will find the cosine and sine of the reference angle:
We must determine the appropriate signs for [latex]x[/latex] and [latex]y[/latex] in the given quadrant. Because our original angle is in the third quadrant, where both [latex]x[/latex] and [latex]y[/latex] are negative, both cosine and sine are negative.

Now we can calculate the [latex](x,y)[/latex] coordinates using the identities [latex]x[/latex] = [latex]cos θ[/latex] and [latex]y[/latex] = [latex]sin θ[/latex]. The coordinates of the point are (-[latex]\frac{\sqrt{3}}{2}[/latex], –[latex]\frac{1}{2}[/latex]) on the unit circle.
Try It #6
Find the coordinates of the point on the unit circle at an angle of [latex]\frac{5π}{3}[/latex].
Media
Access these online resources for additional instruction and practice with sine and cosine functions.
- Trigonometric Functions Using the Unit Circle
- Sine and Cosine from the Unit Circle
- Sine and Cosine from the Unit Circle and Multiples of Pi Divided by Six
- Sine and Cosine from the Unit Circle and Multiples of Pi Divided by Four
- Trigonometric Functions Using Reference Angles
Section Exercises
Verbal
1. Describe the unit circle.
2. What do the x- and y-coordinates of the points on the unit circle represent?
3. Discuss the difference between a coterminal angle and a reference angle.
4. Explain how the cosine of an angle in the second quadrant differs from the cosine of its reference angle in the unit circle.
5. Explain how the sine of an angle in the second quadrant differs from the sine of its reference angle in the unit circle.
Algebraic
For the following exercises, use the given sign of the sine and cosine functions to find the quadrant in which the terminal point determined by [latex]t[/latex] lies.
6. [latex]sin(t)[/latex] < 0 and [latex]cos(t)[/latex] < 0
7. [latex]sin(t)[/latex] > 0 and [latex]cos(t)[/latex] > 0
8. [latex]sin(t)[/latex] > 0 and [latex]cos(t)[/latex] < 0
9. [latex]sin(t)[/latex] < 0 and [latex]cos(t)[/latex] > 0
For the following exercises, find the exact value of each trigonometric function.
10. [latex]sin[/latex] [latex]\frac{π}{2}[/latex]
11. [latex]sin[/latex] [latex]\frac{π}{3}[/latex]
12. [latex]cos[/latex] [latex]\frac{π}{2}[/latex]
13. [latex]cos[/latex] [latex]\frac{π}{3}[/latex]
14. [latex]sin[/latex] [latex]\frac{π}{4}[/latex]
15. [latex]cos[/latex] [latex]\frac{π}{4}[/latex]
16. [latex]sin[/latex] [latex]\frac{π}{6}[/latex]
17. [latex]sin[/latex] [latex]π[/latex]
18. [latex]sin[/latex] [latex]\frac{3π}{2}[/latex]
19. [latex]cos[/latex] [latex]π[/latex]
20. [latex]cos[/latex] 0
21. [latex]cos[/latex] [latex]\frac{π}{6}[/latex]
22. [latex]sin[/latex] 0
Numeric
For the following exercises, state the reference angle for the given angle.
23. 240°
24. −170°
25. 100°
26. −315°
27. 135°
28. [latex]\frac{5π}{4}[/latex]
29. [latex]\frac{2π}{3}[/latex]
30. [latex]\frac{5π}{6}[/latex]
31. –[latex]\frac{11π}{3}[/latex]
32. –[latex]\frac{7π}{4}[/latex]
33. –[latex]\frac{π}{8}[/latex]
For the following exercises, find the reference angle, the quadrant of the terminal side, and the sine and cosine of each angle. If the angle is not one of the special angles on the unit circle, use a calculator and round to three decimal places.
34. 225°
35. 300°
36. 320°
37. 135°
38. 210°
39. 120°
40. 250°
41. 150°
42. [latex]\frac{5π}{4}[/latex]
43. [latex]\frac{7π}{6}[/latex]
44. [latex]\frac{5π}{3}[/latex]
45. [latex]\frac{3π}{4}[/latex]
46. [latex]\frac{4π}{3}[/latex]
47. [latex]\frac{2π}{3}[/latex]
48. [latex]\frac{5π}{6}[/latex]
49. [latex]\frac{7π}{4}[/latex]
For the following exercises, find the requested value.
50. If [latex]cos[/latex] [latex]t[/latex] = [latex]\frac{1}{7}[/latex]and [latex]t[/latex] is in the 4th quadrant, find [latex]sin(t)[/latex].
51. If [latex]cos[/latex] [latex]t[/latex] = [latex]\frac{2}{9}[/latex] and [latex]t[/latex] is in the 1st quadrant, find [latex]sin(t)[/latex].
52. If [latex]sin[/latex] [latex]t[/latex] = [latex]\frac{3}{8}[/latex] and [latex]t[/latex] is in the 2nd quadrant, find [latex]cos(t)[/latex].
53. If [latex]sin[/latex] [latex]t[/latex] = –[latex]\frac{1}{4}[/latex]and [latex]t[/latex] is in the 3rd quadrant, find [latex]cos(t)[/latex].
54. Find the coordinates of the point on a circle with radius 15 corresponding to an angle of 220°.
55. Find the coordinates of the point on a circle with radius 20 corresponding to an angle of 120°.
56. Find the coordinates of the point on a circle with radius 8 corresponding to an angle of [latex]\frac{7π}{4}[/latex].
57. Find the coordinates of the point on a circle with radius 16 corresponding to an angle of [latex]\frac{5π}{9}[/latex].
58. State the domain of the sine and cosine functions.
59. State the range of the sine and cosine functions.
Graphical
For the following exercises, use the given point on the unit circle to find the value of the sine and cosine of [latex]t[/latex].
60.
61.
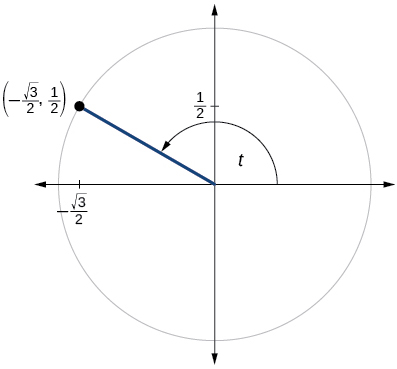
62.
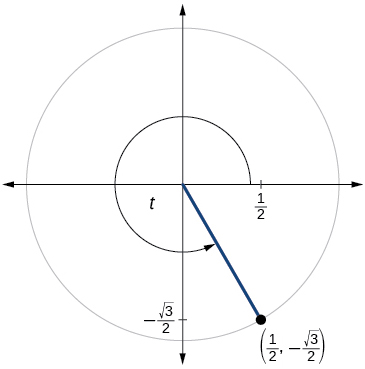
63.
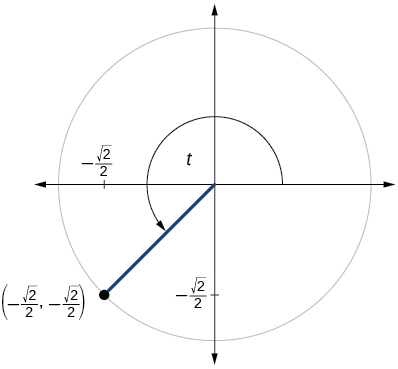
64.

65.
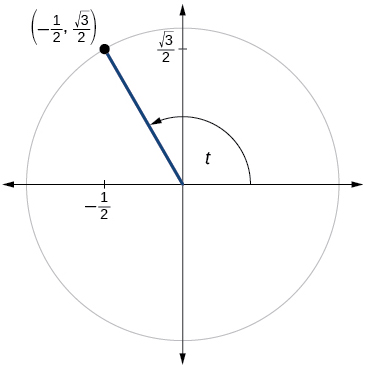
66.

67.
68.
69.
70.
71.
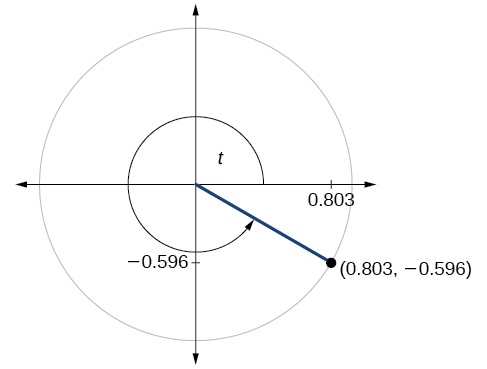
72.
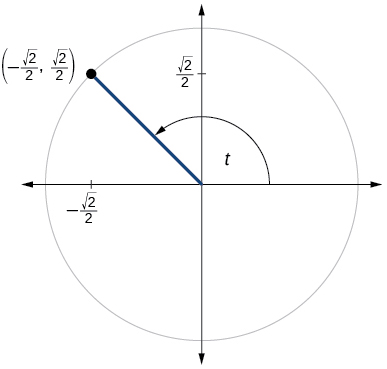
73.
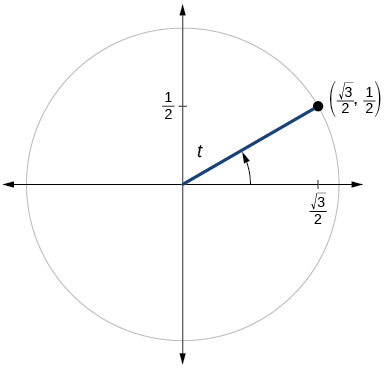
74.
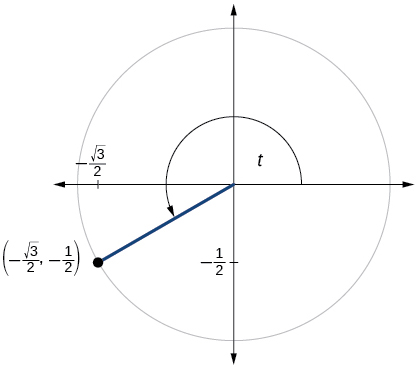
75.
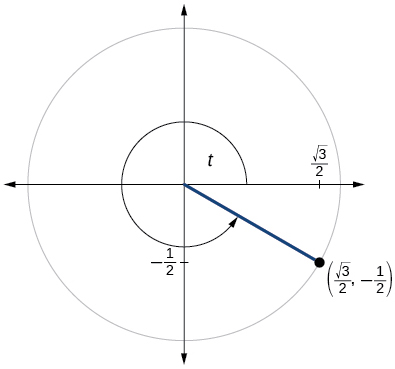
76.

77.
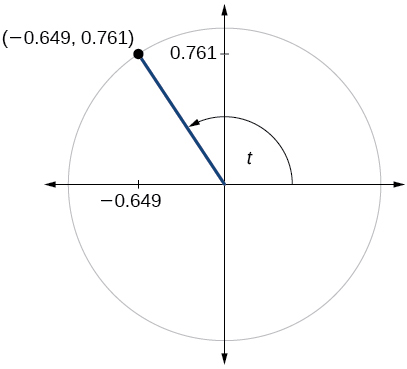
78.
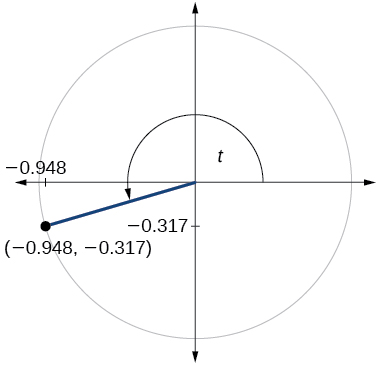
79.
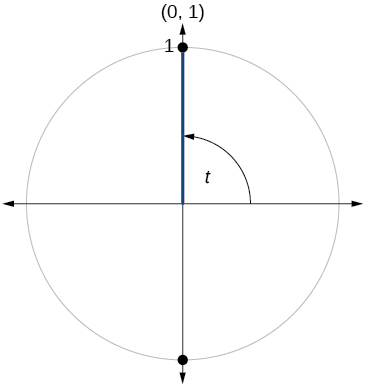
Extensions
For the following exercises, evaluate.
80. [latex]sin[/latex]([latex]\frac{11π}{3}[/latex]) [latex]cos[/latex](-[latex]\frac{5π}{6}[/latex])
81. [latex]sin[/latex]([latex]\frac{3π}{4}[/latex]) [latex]cos[/latex]([latex]\frac{5π}{3}[/latex])
82. [latex]sin[/latex](-[latex]\frac{4π}{3}[/latex]) [latex]cos[/latex](-[latex]\frac{π}{2}[/latex])
83. [latex]sin[/latex](-[latex]\frac{9π}{4}[/latex]) [latex]cos[/latex](-[latex]\frac{π}{6}[/latex])
84. [latex]sin[/latex]([latex]\frac{π}{6}[/latex]) [latex]cos[/latex](-[latex]\frac{π}{3}[/latex])
85. [latex]sin[/latex]([latex]\frac{7π}{4}[/latex]) [latex]cos[/latex](-[latex]\frac{2π}{3}[/latex])
86. [latex]cos[/latex]([latex]\frac{5π}{6}[/latex]) [latex]cos[/latex]([latex]\frac{2π}{3}[/latex])
87. [latex]cos[/latex](-[latex]\frac{π}{3}[/latex]) [latex]cos[/latex]([latex]\frac{π}{4}[/latex])
88. [latex]sin[/latex](-[latex]\frac{5π}{4}[/latex]) [latex]sin[/latex]([latex]\frac{11π}{6}[/latex])
89. [latex]sin[/latex]([latex]π[/latex]) [latex]sin[/latex]([latex]\frac{π}{6}[/latex])
Real-World Applications
For the following exercises, use this scenario: A child enters a carousel that takes one minute to revolve once around. The child enters at the point (0,1), that is, on the due north position. Assume the carousel revolves counterclockwise.
90. What are the coordinates of the child after 45 seconds?
91. What are the coordinates of the child after 90 seconds?
92. What is the coordinates of the child after 125 seconds?
93. When will the child have coordinates (0.707,–0.707) if the ride lasts 6 minutes? (There are multiple answers.)
94. When will the child have coordinates (−0.866,−0.5) if the ride last 6 minutes?

