"

11 Applications and Modeling

Topics Covered

In case you missed something in class, or just want to review a specific topic covered in this Module, here is a list of topics covered:
  1. Use a Problem Solving Strategy for Word Problems
  2. Solve Percent Applications
  3. Solve Simple Interest Applications
  4. Geometry Applications
  5. Solve Formulas for One Variable
  6. Key Concepts

Use a Problem-Solving Strategy for Word Problems

Have you ever had any negative experiences in the past with word problems? When we feel we have no control, and continue repeating negative thoughts, we set up barriers to success. Realize that your negative experiences with word problems are in your past. To move forward you need to calm your fears and change your negative feelings.

Now that we can solve equations, we are ready to apply our new skills to word problems. We will develop a strategy we can use to solve any word problem successfully.

How to use a problem-solving strategy for word problems
  1. Read the problem. Make sure all the words and ideas are understood.
  2. Identify what you are looking for.
  3. Name what you are looking for. Choose a variable to represent that quantity.
  4. Translate into an equation. It may be helpful to restate the problem in one sentence with all the important information. Then, translate the English sentence into an algebra equation.
  5. Solve the equation using proper algebra techniques.
  6. Check the answer in the problem to make sure it makes sense.
  7. Answer the question with a complete sentence.

When dealing with real-world applications, there are certain expressions that we can translate directly into math. The table lists some common verbal expressions and their equivalent mathematical expressions.

Verbal Translation to Math Operations
One number exceeds another by a x, ​x + a
Twice a number 2x
One number is a more than another number x,​ x + a
One number is a less than twice another number x, 2x − a
The product of a number and a, decreased by b ax − b
The quotient of a number and the number plus a is three times the number \displaystyle\frac{x} {x+a} = 3x
The product of three times a number and the number decreased by b is c 3x(x − b) = c

Try it!

Normal yearly snowfall at the local ski resort is 12 inches more than twice the amount it received last season. The normal yearly snowfall is 62 inches. What was the snowfall last season at the ski resort?

Solution

Steps Algebraic
1. Read the problem. NA
2. Identify what you are looking for. What was the snowfall last season?
3. Name what we are looking for and
choose a variable to represent it.		 Let s = the snowfall last season.
4. Translate.
Restate the problem in one sentence with all the important information.		 Step 4 is to translate. Restate the problem in one sentence with all the important information. The normal snowfall is 62, was is the equals sign, and twelve more than twice the amount last year is 2 s plus 12. The equation is 62 is equal to 2 s plus 12
Translate into an equation. The equation is 62 is equal to 2 s plus 12
5. Solve the equation. The equation is 62 is equal to 2 s plus 12
Subtract 12 from each side. The equation is 62 minus 12 is equal to 2 s plus 12 minus 12.
Simplify. Fifty equals 2s.
Divide each side by two. 50 divided by 2 equals 2s divided by 2.
Simplify. 25 equals s.
6. Check: First, is our answer reasonable?

Yes, having 25 inches of snow seems OK.
The problem says the normal snowfall is twelve inches more than twice the number of last season. Twice 25 is 50 and 12 more than that is 62.
7. Answer the question. The snowfall last season was 25 inches.

2. A married couple together earns $110,000 a year. The wife earns $16,000 less than twice what her husband earns. What does the husband earn?

Solution

Steps Algebraic
1. Read the problem. NA
2. Identify what you are looking for. How much does the husband earn?
3. Name.

Choose a variable to represent the amount the husband earns.

The wife earns $16,000 less than twice that.

  Let h = the amount the husband earns.
4. Translate.

Restate the problem in one sentence with all the important information. Translate into an equation.

 2h − 16,000 = the amount the wife earns.

Together the husband and wife earn $110,000.
5. Solve the equation.

Combine like terms.

Add 16,000 to both sides and simplify.

Divide each side by three.

 

 h + 2h − 16,000 = 110,000

h + 2h − 16,000 = 110,000

3h − 16,000 = 110,000

3h = 126,000

h = 42,000

$42,000 amount husband earns

2h − 16,000 amount wife earns

2(42,000) − 16,000

84,000 − 16,000

68,000
6. Check:

If the wife earns $68,000 and the husband earns $42,000, is that $110,000?

 Yes!
7. Answer the question. The husband earns $42,000 a year

Access this online resource for additional instruction and practice with using a problem-solving strategy.

Solve Percent Applications

How many cents are in one dollar? There are 100 cents in a dollar. How many years are in a century? There are 100 years in a century. Does this give you a clue about what the word “percent” means? It is really two words, “per cent,” and means per one hundred. A percent is a ratio whose denominator is 100. We use the percent symbol %, to show percent.

A percent is a ratio whose denominator is 100.

Similarly, 25% means a ratio of \displaystyle\frac{25}{100}, 3% means a ratio of \displaystyle\frac{3}{100}, and 100% means a ratio of \displaystyle\frac{100}{100}. In words, “one hundred percent” means the total 100% is \displaystyle\frac{100}{100}, and since \displaystyle\frac{100}{100} = 1, we see that 100% means 1 whole.
There are several methods to solve percent equations. In algebra, it is easiest if we just translate English sentences into algebraic equations and then solve the equations. Be sure to change the given percent to a decimal before you use it in the equation.

How to convert a percent number to a decimal number

To convert a percent number to a decimal number, we move the decimal point two places to the left and remove the % sign. (Sometimes the decimal point does not appear in the percent number, but just like we can think of the integer 6 as 6.0, we can think of 6% as 6.0%. Notice that we may need to add zeros in front of the number when moving the decimal to the left.

  1. Write the percent as a ratio with the denominator 100.
  2. Convert the fraction to a decimal by dividing the numerator by the denominator.

Try it!

  1. Convert each percent to a decimal:

a. 135%

b. 12.5%

    Solution

    a.

    Steps Algebraic
    Example 135%
    Write as a ratio with denominator 100. \displaystyle\frac{135}{100}
    Change the fraction to a decimal by dividing the numerator by the denominator. 1.35

    b.

    Steps Algebraic
    Example 12.5%
    Write as a ratio with denominator 100. \displaystyle\frac{12.5}{100}
    Change the fraction to a decimal by dividing the numerator by the denominator. 0.125

    2. Translate and solve:

    a. What number is 45% of 84?
    b. 8.5% of what amount is $4.76?

    Solution

    a.

    Steps Algebraic
    Example What number is 45% of 84? Translate into algebra.
    Translate into algebra. Let n = the number. Write n as is equal to 0.45 times 84.
    Multiply. The result is n is equal 37.8. 37.8 is 45 percent of 84.
    Solution 37.8 is 45% of 84.

    b.

    Steps Algebraic
    Example What number is 45% of 84? Translate into algebra.
    Translate. Let n = the amount. .085 times n equals 4.76
    Multiply. .085n equals 4.76
    Divide both sides by 0.085 and simplify. n = 56
    Solution 8.5% of $56 is $4.76

    Now that we have a problem-solving strategy to refer to, and have practiced solving basic percent equations, we are ready to solve percent applications. Be sure to ask yourself if your final answer makes sense—since many of the applications we will solve involve everyday situations, you can rely on your own experience.

    Try it!

    1. The label on Audrey’s yogurt said that one serving provided 12 grams of protein, which is 24% of the recommended daily amount. What is the total recommended daily amount of protein?

    Solution

    Steps Algebraic
    What are you asked to find? What total amount of protein is recommended?
    Choose a variable to represent it. Let a = total amount of protein.
    Write a sentence that gives the
    information to find it.    		 12 grams is 24% of the total amount.
    Translate into an equation. 12 equals .24 times a
    Solve. 50 equals a
    Check: Does this make sense? Yes, 24% is about \displaystyle\frac{1}{4} of the total and

     
    12 is about \displaystyle\frac{1}{4} of 50.
    Write a complete sentence to answer the question. The amount of protein that is recommended is 50g.

    2. Veronica is planning to make muffins from a mix. The package says each muffin will be 240 calories and 60 calories will be from fat. What percent of the total calories is from fat?

    Solution

    Steps Algebraic
    What are you asked to find? What percent of the total calories is fat?
    Choose a variable to represent it. Let  p = percent of fat.
    Write a sentence that gives the
    information to find it.    		 Write a sentence that gives the information to find it. What percent of 240 is 60?
    Translate the sentence into an equation. Translate the sentence into an equation. The equation is p times 240 is equal to 60.
    Multiply. Multiply. The result is 240 p is equal to 60.
    Divide both sides by 240. Divided both sides by 240. The result is p is equal to 0.25.
    Put in percent form. Put it in percent form. The result is p is equal to 25 percent.
    Check: does this make sense?
    		 Yes, 25% is one-fourth; 60 is one-fourth of 240. So, 25% makes sense.
    Write a complete sentence to answer the question. Of the total calories in each muffin, 25% is fat.

    When you buy an item on sale, the original price has been discounted by some dollar amount. The discount rate, usually given as a percent, is used to determine the amount of the discount. To determine the amount of discount, we multiply the discount rate by the original price.

    The price a retailer pays for an item is called the original cost. The retailer then adds a mark-up to the original cost to get the list price, the price he sells the item for. The mark-up is usually calculated as a percent of the original cost. To determine the amount of mark-up, multiply the mark-up rate by the original cost.

    Discount
    amount of discount = discount rate · original price
    sale price = original amount – discount price

    The sale price should always be less than the original price.

    Mark-up
    amount of mark-up = mark-up rate · original price
    list price = original cost + mark-up

    The list price should always be more than the original cost.

    Try it!

    Liam’s art gallery bought a painting at an original cost of $750. Liam marked the price up 40%. Find a. the amount of mark-up and b. the list price of the painting.

    Solution

    a.

    Steps Algebraic
    Identify what you are asked to find and choose a variable to represent it. What is the amount of mark-up?
    Let m = the amount of mark-up.
    Write a sentence that gives the
    information to find it.    		 Write a sentence that gives the information to find it. The mark-up is 40 percent of the 750 dollar original cost.
    Translate into an equation. Translate into an equation. . The equation is m is equal to 0.40 times 750.
    Solve the equation. m = 300
    Write a complete sentence. The mark-up on the painting was $300.

    b.

    Steps Algebraic
    Identify what you are asked to find and choose a variable to represent it. What is the list price?
    Let p = the list price.
    Write a sentence that gives the
    information to find it.    		 What is the list price? Let p be equal to the list price. Write a sentence that gives the information to find it. The list price is original cost plus the mark-up.
    Translate into an equation. Translate into an equation. The result is p is equal to 750 plus 300.
    Solve the equation. Solve the equation. The result is p is equal to 1050.
    Check. Is the list price more than the original cost?
    Is $1,050 more than $750? Yes.
    Write a complete sentence. The list price of the painting was $1,050.

    Solve Simple Interest Applications

    Interest is a part of our daily lives. From the interest earned on our savings to the interest we pay on a car loan or credit card debt, we all have some experience with interest in our lives.

    The amount of money you initially deposit into a bank is called the principal, P, and the bank pays you interest, I. When you take out a loan, you pay interest on the amount you borrow, also called the principal.

    In either case, the interest is computed as a certain percent of the principal, called the rate of interest, r. The rate of interest is usually expressed as a percent per year and is calculated by using the decimal equivalent of the percent. The variable t, (for time) represents the number of years the money is saved or borrowed.

    Interest is calculated as simple interest or compound interest. Here we will use simple interest.

    Simple Interest

    If an amount of money, P, called the principal, is invested or borrowed for a period of t years at an annual interest rate r, the amount of interest, I, earned or paid is

     I = Prt, 

    Where

    		 I = interest
    P = principal
    r = rate
    t = time

    Interest earned or paid according to this formula is called simple interest.

    The formula we use to calculate interest is I = Prt. To use the formula, we substitute in the values for variables that are given, and then solve for the unknown variable. It may be helpful to organize the information in a chart.

    Try it!

    Areli invested a principal of $950 in her bank account that earned simple interest at an interest rate of 3%. How much interest did she earn in five years?

    Solution

    I = ?

    P = $950

    r = 3%

    t = 5 years

    Steps Algebraic
    Identify what you are asked to find and choose a variable to represent it. What is the simple interest?

    Let I = interest.
    Write the formula. I = Prt
    Substitute in the given information. I = (950)(0.03)(5)
    Simplify. I = 142.5
    Check. Is $142.50 a reasonable amount of interest on $950?

    Yes.
    Write a complete sentence. The interest is $142.50.

    There may be times when we know the amount of interest earned on a given principal over a certain length of time, but we do not know the rate.

    Try it!

    Hang borrowed $7,500 from her parents to pay her tuition. In five years, she paid them $1,500 interest in addition to the $7,500 she borrowed. What was the rate of simple interest?

    Solution

    I = $1500

    P = $7500

    r = ?

    t = 5 years

    Steps Algebraic
    Identify what you are asked to find and choose a variable to represent it. What is the rate of simple interest?

    Let r = rate of interest.
    Write the formula. I = Prt
    Substitute in the given information. 1,500 = (7,500)r(5)
    Multiply. 1,500 = 37,500r
    Divide. 0.04 = r
    Change to percent form. 4% = r
    Check. I = Prt
    1,500 \displaystyle\stackrel{?}{=} (7,500)(0.04)(5)
    1,500 = 1,500✓
    Write a complete sentence. The rate of interest was 4%.

    In the next example, we are asked to find the principal—the amount borrowed.

    Try it!

    Sean’s new car loan statement said he would pay $4,866.25 in interest from a simple interest rate of 8.5% over five years. How much did he borrow to buy his new car?

    Solution

    I = 4,866.25

    P = ?

    r = 8.5%

    t = 5years

    Steps Algebraic
    Identify what you are asked to find and choose a variable to represent it. What is the amount borrowed (the principal)?

    Let P = principal borrowed.
    Write the formula. I = Prt
    Substitute in the given information. 4,866.25 = P(0.085)(5)
    Multiply. 4,866.25 = 0.425P
    Divide. 11,450 = P
    Check. I = Prt
    4,866.25 \displaystyle\stackrel{?}{=} (11,450)(0.085)(5)
    4,866.25 = 4,866.25✓
    Write a complete sentence. The principal was $11,450.

    Geometry Applications

    In this objective we will use some common geometry formulas. We will adapt our problem-solving strategy so that we can solve geometry applications. The geometry formula will name the variables and give us the equation to solve.

    In addition, since these applications will all involve shapes of some sort, most people find it helpful to draw a figure and label it with the given information. We will include this in the first step of the problem-solving strategy for geometry applications.

    When we solve geometry applications, we often have to use some of the properties of the figures. We will review those properties as needed.

    The next example involves the area of a triangle. The area of a triangle is one-half the base times the height. We can write this as \displaystyle A=\frac{1}{2}bh, where b = length of the base and h = height.The figure is a triangle with its height shown. Its base is b and its height is h. The formula for the area of the triangle is A is equal to one-half times b times h.

    Try it!

    The area of a triangular painting is 126 square inches. The base is 18 inches. What is the height?

    Solution

    Steps Algebraic
    1. Read the problem. NA
    2. Identify what you are looking for. height of a triangle
    3. Name.
    Choose a variable to represent it.
    Draw the figure and label it with the given information.    		 Let h = the height.
    Area = 126 sq. in.
    The figure is a triangle with its height shown as h. Its base is b 18 in.
    4. Translate.
    Write the appropriate formula.
    Substitute in the given information.    		 \displaystyle A = \frac{1}{2}bh
    \displaystyle 126 = \frac{1}{2}\cdot 18\cdot h
    5. Solve the equation.
    Divide both sides by 9.    		 126 = 9h
    14 = h
    6. Check.


    		 \displaystyle A = \frac{1}{2}bh
    \displaystyle 126 = \frac{1}{2}\cdot 18\cdot 14
    126=126✓
    7. Answer the question. The height of the triangle is 14 inches.

    In the next example, we will work with a right triangle. To solve for the measure of each angle, we need to use two triangle properties. In any triangle, the sum of the measures of the angles is 180°. We can write this as a formula: m∠A + m ∠B + m ∠C=180. Also, since the triangle is a right triangle, we remember that a right triangle has one 90° angle.

    Here, we will have to define one angle in terms of another. We will wait to draw the figure until we write expressions for all the angles we are looking for.

    Try it!

    The measure of one angle of a right triangle is 40 degrees more than the measure of the smallest angle. Find the measures of all three angles.

    Solution

    Steps Algebraic
    1. Read the problem. NA
    2. Identify what you are looking for. the measures of all three angles
    3. Name. Choose a variable to represent it.


    		 Let a = 1st angle.
    a + 40 = 2nd angle
    90 = 3rd angle (the right angle)
    Draw the figure and label it with the given information. 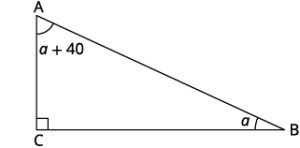The figure is the triangle A B C, with A measuring a plus 40, B measuring a, and C measuring 90.
    4. Translate.
    Write the appropriate formula. m∠A + m∠B + m∠C = 180
    Substitute into the formula. a (a + 40) + 90 = 180
    5. Solve the equation.






    		 2a + 130 = 180
    2a = 50
    a = 25 first angle
    a + 40 second angle
    25 + 40
    65
    90 third angle
    6. Check.

    		 25 + 65 + 90 \displaystyle\stackrel{?}{=} 180
    180 = 180✓
    7. Answer the question. The three angles measure 25°, 65°, and 90°.

    The next example uses another important geometry formula. The Pythagorean Theorem tells how the lengths of the three sides of a right triangle relate to each other. Writing the formula in every exercise and saying it aloud as you write it may help you memorize the Pythagorean Theorem.

    The Pythagorean Theorem

    In any right triangle, where a and b are the lengths of the legs, and c is the length of the hypotenuse, the sum of the squares of the lengths of the two legs equals the square of the length of the hypotenuse.

    The figure is a right triangle with sides a and b, and a hypotenuse c. a squared plus b squared is equal to c squared. In a right triangle, the sum of the squares of the lengths of the two legs equals the square of the length of the hypotenuse.

    Try it!

    Use the Pythagorean Theorem to find the length of the other leg in:

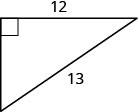
    This figure is a right triangle with one leg that is 12 units and a hypotenuse that is 13 units.

    Solution

    Steps Algebraic
    1. Read the problem. NA
    2. Identify what you are looking for. the length of the leg of the triangle
    3. Name.
    Choose a variable to represent it. Let a = the leg of the triangle.
    Label side a. 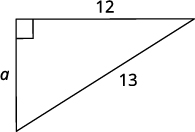Now the figure is a right triangle with one leg that is 12 units, one leg that is a, and a hypotenuse that is 13 units.
    4. Translate. NA
    Write the appropriate formula.
    Substitute.

    a2 + b2 = c2

    a2 + 122 = 132
    5. Solve the equation.
    Isolate the variable term.
    Use the definition of square root.
    Simplify.

    a2  + 144 = 169

    a2 = 25

    a = √25

    a = 5
    6. Check.
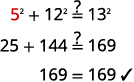    		 Step 6 is to check the answer. Is 5 squared plus 12 squared is equal to 13 squared? Is 25 plus 144 equal to 169? 169 is equal to 169. The solution checks.
    7. Answer the question. The length of the leg is 5.

    The next example is about the perimeter of a rectangle. Since the perimeter is just the distance around the rectangle, we find the sum of the lengths of its four sides—the sum of two lengths and two widths. We can write is as P = 2L + 2W where L is the length and W is the width. To solve the example, we will need to define the length in terms of the width.

    Try it!

    The length of a rectangle is six centimeters more than twice the width. The perimeter is 96 centimeters. Find the length and width.

    Solution

    Steps Algebraic
    1. Read the problem. NA
    2. Identify what we are looking for. the length and the width
    3. Name. Choose a variable to represent the width.

    The length is six more than twice the width.
    Let w = width.
    2w + 6 = length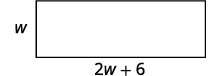The figure is a rectangle with the width labeled w, a length labeled 2 w plus 6, and the perimeter labeled P is equal to 96 centimeters.
    P = 96 cm
    4. Translate. NA
    Write the appropriate formula. P = 2L + 2W
    Substitute in the given information. 96 = 2(2w + 6) + 2w
    5. Solve the equation.

    96 = 4w + 12 + 2w

    96 = 6w + 12

    84 = 6w

    14 = W (width)

    Length: 2w + 6

    2(14) + 6

    34 = length
    6. Check.

    The figure is a rectangle with its width labeled 14 centimeters and its length labeled 34 centimeters. The perimeter is given by P is equal to 2 L plus 2 W.

    P = 2L + 2W

    96 \displaystyle\stackrel{?}{=} 2 · 34 + 2 · 14

    96 = 96✓
    7. Answer the question. The length is 34 cm and the width is 14 cm.

    The next example is about the perimeter of a triangle. Since the perimeter is just the distance around the triangle, we find the sum of the lengths of its three sides. We can write this as P = a + b + c, where a, b, and c are the lengths of the sides.

    Try it!

    1. One side of a triangle is three inches more than the first side. The third side is two inches more than twice the first. The perimeter is 29 inches. Find the length of the three sides of the triangle.

    Solution

    Steps Algebraic
    1. Read the problem. NA
    2. Identify what we are looking for. the lengths of the three sides of a triangle
    3. Name. Choose a variable to represent the length of the first side.


    		 Let x = length of 1st side.
    x + 3 = length of 2nd side
    2x + 2 = length of 3rd side
    The figure is a triangle with sides labeled x, x plus 3, and 2 x plus 2, and a perimeter shown to be 29 inches.
    4. Translate.
    Write the appropriate formula.
    Substitute in the given information.

    		 P = a + b + c
    29 = x + (x + 3) + (2x + 2)
    5. Solve the equation.






    		 29 = 4x + 5
    24 = 4x
    6 = x length of first side 
    x + 3 length of second side 
    6 + 3 = 9
    2x + 2 length of third side 
    2 \displaystyle\cdot 6 + 2 = 14 
    6. Check.
    The figure is a triangle with sides labeled, 6, 9, and 14. Is 29 equal to 6 plus 9 plus 14? 29 is equal to 29, so the answers check.

    		 29 \displaystyle\stackrel{?}{=} 6 + 9 + 14 
    29 = 29✓
    7. Answer the question. The lengths of the sides of the triangle
    are 6, 9, and 14 inches.

    2. The perimeter of a rectangular soccer field is 360 feet. The length is 40 feet more than the width. Find the length and width.

    The figure is an illustration of rectangular soccer field.

    Solution

    Steps Algebraic
    1. Read the problem. NA
    2. Identify what we are looking for. the length and width of the soccer field
    3. Name. Choose a variable to represent it.
    The length is 40 feet more than the width.
    Draw the figure and label it with the given information.    		 Let w = width.
    w + 40 = length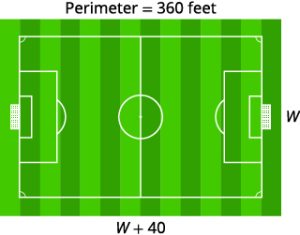

    The figure is an illustration of a rectangular soccer field, its length labeled w and its width labeled w plus 40, and its perimeter given as 360 feet.
    4. Translate.
    Write the appropriate formula and substitute.

    		 P = 2L + 2W
    360 = 2(w + 40) + 2w
    5. Solve the equation. 360 = 2w + 80 + 2w

        360 = 4w + 80

        280 = 4w

    70 = w the width of the field

    w + 40 the length of the field

        70 + 40 = 110
    6. Check.

    P = 2L + 2W

    360 \displaystyle\stackrel{?}{=} 2(110) + 2(70)

    360 = 360✓
    7. Answer the question. The length of the soccer field is 110 feet and the width is 70 feet.

    Applications of these geometric properties can be found in many everyday situations as shown in the next example.

    Try it!

    Kelvin is building a gazebo and wants to brace each corner by placing a 10” piece of wood diagonally as shown.

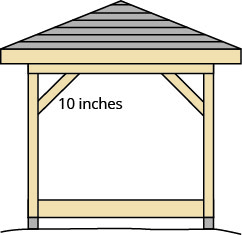    The figure is an illustration of a gazebo whose corner forms a right triangle with a 10 inch piece of wood that is placed diagonally to brace it.How far from the corner should he fasten the wood if he wants the distances from the corner to be equal? Approximate to the nearest tenth of an inch.

    Solution

    Steps Algebraic
    1. Read the problem. NA
    2. Identify what we are looking for. the distance from the corner that the
    bracket should be attached
    3. Name. Choose a variable to represent it.

    Draw the figure and label it with the given information.

    		 Let x = the distance from the corner.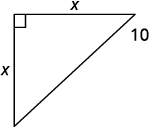
    The figure is right triangle with both sides labeled x and a hypotenuse labeled 10.
    4. Translate.
    Write the appropriate formula and substitute.    		  a2 + b2 = c2

    x2  + x2 = 102

    5. Solve the equation.
    Isolate the variable.
    Use the definition of square root.
    Simplify. Approximate to the nearest tenth.    		 2x2 = 100

        x2 = 50

        x = √50

        x ≈ 7.1
    6. Check.
    		 a2  + b2 = c2

    (7.1)2 + (7.1)2 ≈ 102  Yes.
    7. Answer the question. Kelvin should fasten each piece of wood approximately 7.1” from the corner.

    Access this online resource for additional instruction and practice with solving for a variable in literal equations.

     

    Solve Formulas for One Variable

    We have all probably worked with some geometric formulas in our study of mathematics. Formulas are used in so many fields, it is important to recognize formulas and be able to manipulate them easily.

    It is often helpful to solve a formula for a specific variable. If you need to put a formula in a spreadsheet, it is not unusual to have to solve it for a specific variable first. We isolate that variable on one side of the equals sign with a coefficient of one and all other variables and constants are on the other side of the equal sign.

    Geometric formulas often need to be solved for another variable, too. The formula \displaystyle V=\frac{1}{3}πr^{2}h is used to find the volume of a right circular cone when given the radius of the base and height. In the next example, we will solve this formula for the height.

    Try it!

    Solve the formula V = \displaystyle \frac{1}{3}πr2h for h.

    Solution

    Steps Algebraic
    Write the formula. \displaystyle V=\frac{1}{3}πr^{2}h
    Remove the fraction on the right. 3 • V= 3•\displaystyle \frac{1}{3}πr2h
    Simplify. 3V = πr2h
    Divide both sides by πr2. \displaystyle\frac{3V}{\pi r^{2}}=h

    We could now use this formula to find the height of a right circular cone when we know the volume and the radius of the base, by using the formula h = \displaystyle \frac{3V}{\pi r^{2}} .

    In the sciences, we often need to change temperature from Fahrenheit to Celsius or vice versa. If you travel in a foreign country, you may want to change the Celsius temperature to the more familiar Fahrenheit temperature.

    Try it!

    Solve the formula \displaystyle C=\frac{5}{9}(F-32) for F.

    Solution

    Steps Algebraic
    Write the formula. Write the formula. It is C is equal to five-ninths times the quantity F minus 32.
    Remove the fraction on the right. Clear the fractions by multiplying each side: nine-fifths C is equal to nine-fifths time s five-ninths times the quantity F minus 32.
    Simplify. Simplify. The result is nine-fifths C is equal to F minus 32.
    Add 32 to both sides. Add 32 to both sides. The result is nine-fifths C plus 32 is equal to F. We can now use the formula F is equal to nine-fifths C plus 32 to find the Fahrenheit temperature when we know the Celsius.

    We can now use the formula \displaystyle F=\frac{9}{5}C+32 to find the Fahrenheit temperature when we know the Celsius temperature.

    The next example uses the formula for the surface area of a right cylinder.

    Try it!

    Solve the formula S = 2πr2 + 2πrh for h.

    Solution

    Steps Algebraic
    Write the formula. S = 2πr2 + 2πrh
    Isolate the h term by subtracting 2πr2 from each side. S – 2πr2 = 2πr2 – 2πr2 + 2πrh
    Simplify. S – 2πr2 = 2πrh
    Solve for h by dividing both sides by 2πr. \displaystyle \frac{S-2\pi r^{2}}{2\pi r}= \frac{2\pi rh}{2\pi r}
    Simplify. \displaystyle \frac{S-2\pi r^{2}}{2\pi r}=h

    Key Concepts

    • How To Use a Problem-Solving Strategy for Word Problems
      1. Read the problem. Make sure all the words and ideas are understood.
      2. Identify what you are looking for.
      3. Name what you are looking for. Choose a variable to represent that quantity.
      4. Translate into an equation. It may be helpful to restate the problem in one sentence with all the important information. Then, translate the English sentence into an algebra equation.
      5. Solve the equation using proper algebra techniques.
      6. Check the answer in the problem to make sure it makes sense.
      7. Answer the question with a complete sentence.
    • How To Find Percent Change
      1. Find the amount of change
        change = new amount − original amount
      2. Find what percent the amount of change is of the original amount.
        change is what percent of the original amount?
    • Discount
      amount of discount = discount rate \displaystyle\cdot original price

    sale price = original amount − discount

    • Mark-up
      amount of mark-up = mark-up rate \displaystyle\cdot original cost

    list price = original cost + mark up

    • Simple Interest
      If an amount of money, P, called the principal, is invested or borrowed for a period of t years at an annual interest rate r, the amount of interest, I, earned or paid is:
    I = interest
     I = Prt where P = principal
    r = rate
    t = time
    definition

    License

    Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

    NCB 0502 - Support for Corequisite Algebra Copyright © by Elizabeth Pople is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.

    Share This Book